Expressing numbers in exponential form can simplify complex calculations and provide a deeper understanding of mathematical concepts. One such number is 4√7, which can be rewritten in exponential form. In this article, we will delve into the world of exponents and explore the exponential form of 4√7.
Understanding Exponents and Roots

Exponents and roots are fundamental concepts in mathematics, allowing us to represent repeated multiplication and division operations in a concise manner. An exponent is a small number that is raised to a power, indicating how many times the base number should be multiplied by itself. For instance, 2^3 represents 2 multiplied by itself three times, resulting in 8.
On the other hand, roots represent the inverse operation of exponents. The nth root of a number is a value that, when raised to the power of n, yields the original number. In the case of 4√7, the 4th root of 7 is a value that, when raised to the power of 4, equals 7.
Exponential Form of 4√7
To express 4√7 in exponential form, we can utilize the following rule:
a^(1/n) = nth root of a
where a is the base number and n is the root.
Applying this rule to 4√7, we get:
4√7 = 7^(1/4)
In this exponential form, 7 is the base number, and 1/4 is the exponent.
Working with Exponential Forms

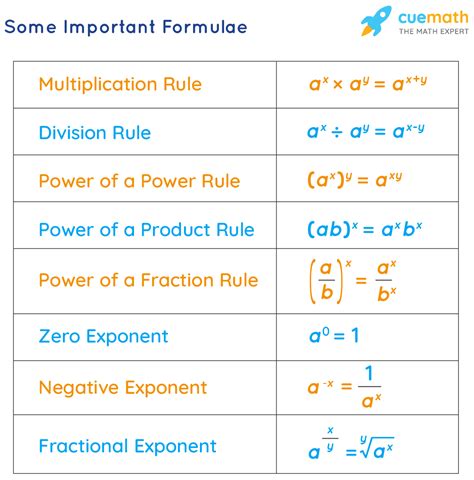
Exponential forms can be manipulated using various rules and properties, allowing us to simplify complex expressions. Here are a few essential rules to keep in mind:
- Product of Powers: When multiplying two exponential expressions with the same base, we add the exponents.
a^m × a^n = a^(m+n)
- Power of a Power: When raising an exponential expression to another power, we multiply the exponents.
(a^m)^n = a^(m×n)
- Quotient of Powers: When dividing two exponential expressions with the same base, we subtract the exponents.
a^m ÷ a^n = a^(m-n)
Using these rules, we can simplify expressions involving exponential forms like 7^(1/4).
Benefits of Exponential Forms
Expressing numbers in exponential form offers several advantages:
- Simplified Calculations: Exponential forms can simplify complex calculations by reducing the number of operations required.
- Clearer Understanding: Exponential forms provide a deeper understanding of mathematical concepts, allowing us to visualize and manipulate expressions more effectively.
- Easy Pattern Recognition: Exponential forms enable us to recognize patterns and relationships between numbers, making it easier to solve problems and make predictions.
Real-World Applications of Exponential Forms

Exponential forms have numerous real-world applications across various fields, including:
- Finance: Exponential forms are used to calculate compound interest rates, investment returns, and loan repayments.
- Science: Exponential forms are used to model population growth, chemical reactions, and physical phenomena like radioactive decay.
- Computer Science: Exponential forms are used in algorithms for solving problems related to graph theory, network analysis, and cryptography.
Common Mistakes to Avoid
When working with exponential forms, it's essential to avoid common mistakes:
- Incorrect Exponentiation: Make sure to raise the correct base to the correct power.
- Inconsistent Units: Ensure that the units of measurement are consistent when working with exponential forms.
- Ignoring Negative Exponents: Don't forget to consider negative exponents, as they can significantly impact the result.
Conclusion: Unlocking the Power of Exponential Forms

In conclusion, expressing 4√7 in exponential form as 7^(1/4) offers a deeper understanding of mathematical concepts and simplifies complex calculations. By mastering the rules and properties of exponential forms, we can unlock a world of possibilities in mathematics and real-world applications.
We encourage you to share your thoughts and questions about exponential forms in the comments section below. What are some common challenges you face when working with exponential forms? How do you apply exponential forms in your field of study or profession? Let's discuss!
FAQ Section:
What is the exponential form of 4√7?
+The exponential form of 4√7 is 7^(1/4).
What are the benefits of using exponential forms?
+Exponential forms simplify complex calculations, provide a clearer understanding of mathematical concepts, and enable easy pattern recognition.
What are some real-world applications of exponential forms?
+Exponential forms have applications in finance, science, and computer science, including compound interest rates, population growth models, and algorithm design.