Convert Two Points To Standard Form Easily

Quick Links :

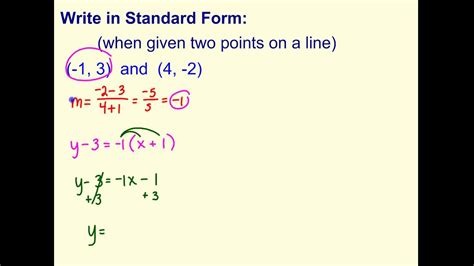
Converting two points to standard form is a fundamental concept in mathematics, particularly in geometry and algebra. It's a crucial skill to master, as it allows us to represent and analyze geometric shapes and equations in a more manageable and standardized way. In this article, we'll delve into the world of standard form, exploring its importance, benefits, and providing a step-by-step guide on how to convert two points to standard form with ease.

What is Standard Form?

Standard form is a way of expressing linear equations, geometric shapes, and other mathematical concepts in a consistent and simplified manner. In the context of two points, standard form refers to the equation of a line that passes through those two points. This equation is typically represented in the form Ax + By = C, where A, B, and C are integers, and x and y are variables.

Why is Standard Form Important?

Standard form is essential in mathematics and real-world applications for several reasons:

• Simplification: Standard form simplifies complex equations and geometric shapes, making it easier to analyze and solve problems.
• Consistency: Standard form provides a consistent way of representing mathematical concepts, allowing for easier comparison and manipulation of equations.
• Efficient problem-solving: Standard form enables efficient problem-solving, as it allows us to quickly identify key characteristics of equations and geometric shapes.

Benefits of Converting Two Points to Standard Form

Converting two points to standard form offers several benefits, including:

• Improved understanding: Standard form helps us understand the relationship between the two points and the line that passes through them.
• Easier graphing: Standard form makes it easier to graph lines and other geometric shapes, as it provides a clear and consistent representation of the equation.
• Enhanced problem-solving: Standard form enables us to solve problems more efficiently, as it allows us to quickly identify key characteristics of equations and geometric shapes.

Step-by-Step Guide to Converting Two Points to Standard Form

Converting two points to standard form is a straightforward process that involves the following steps:

1. Find the slope: Calculate the slope of the line that passes through the two points using the formula m = (y2 - y1) / (x2 - x1).
2. Determine the equation: Use the point-slope form of a linear equation, y - y1 = m(x - x1), to determine the equation of the line.
3. Simplify the equation: Simplify the equation by combining like terms and eliminating any fractions.
4. Write in standard form: Write the equation in standard form by rearranging the terms to match the format Ax + By = C.

Example Problem: Converting Two Points to Standard Form

Suppose we have two points, (2, 3) and (4, 5). To convert these points to standard form, we follow the steps outlined above:

1. Find the slope: Calculate the slope of the line that passes through the two points using the formula m = (y2 - y1) / (x2 - x1) = (5 - 3) / (4 - 2) = 1.
2. Determine the equation: Use the point-slope form of a linear equation, y - y1 = m(x - x1), to determine the equation of the line. Plugging in the values, we get y - 3 = 1(x - 2).
3. Simplify the equation: Simplify the equation by combining like terms and eliminating any fractions. This gives us y - 3 = x - 2.
4. Write in standard form: Write the equation in standard form by rearranging the terms to match the format Ax + By = C. This gives us x - y = -1.

Practical Applications of Converting Two Points to Standard Form

Converting two points to standard form has numerous practical applications in various fields, including:

• Computer graphics: Standard form is used to represent geometric shapes and equations in computer graphics, allowing for efficient rendering and manipulation of images.
• Engineering: Standard form is used to analyze and design systems, such as bridges and buildings, by representing the equations of motion and stress in a standardized way.
• Physics: Standard form is used to represent the equations of motion and energy in physics, allowing for efficient problem-solving and analysis.

Common Challenges and Solutions

When converting two points to standard form, you may encounter several challenges, including:

• Difficulty finding the slope: If the two points have the same x-coordinate or the same y-coordinate, the slope will be undefined or zero. In such cases, use the equation x = x1 or y = y1 to represent the line.
• Simplifying the equation: If the equation is complex or has fractions, simplify it by combining like terms and eliminating any fractions.

To overcome these challenges, follow these solutions:

• Use alternative methods: If you're having trouble finding the slope, use alternative methods, such as the equation x = x1 or y = y1.
• Break down the equation: If the equation is complex, break it down into smaller parts, and simplify each part separately.

We hope this comprehensive guide has helped you understand the importance of converting two points to standard form. With practice and patience, you'll become proficient in converting two points to standard form, unlocking a world of mathematical possibilities.

Take a moment to share your thoughts on this article. How do you think converting two points to standard form can be applied in real-world scenarios? What challenges have you faced when converting two points to standard form, and how did you overcome them? Share your experiences and insights in the comments below.