Right angles are a fundamental concept in geometry, and understanding how two lines form a right angle is essential for various mathematical and real-world applications. In this article, we will explore six ways two lines can form a right angle, including definitions, examples, and practical applications.
What is a Right Angle?

A right angle is an angle whose measure is exactly 90 degrees. It is formed when two lines intersect, and the resulting angle is equal to 90 degrees. Right angles are denoted by a small square symbol (⊥) and are commonly used in various mathematical and real-world applications, such as architecture, engineering, and design.
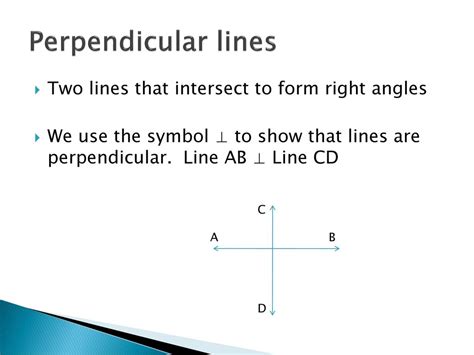
1. Perpendicular Lines

One way two lines can form a right angle is when they are perpendicular to each other. Perpendicular lines are lines that intersect at a right angle, forming a 90-degree angle. For example, the x-axis and y-axis in a coordinate plane are perpendicular to each other, forming a right angle.
Example: Coordinate Plane
In a coordinate plane, the x-axis and y-axis intersect at a point called the origin (0, 0). The x-axis and y-axis are perpendicular to each other, forming a right angle. This right angle is essential for graphing functions and equations in mathematics.
2. Intersecting Lines with a 90-Degree Angle

Another way two lines can form a right angle is when they intersect at a 90-degree angle. This can occur when two lines intersect at a point, and the resulting angle is equal to 90 degrees. For example, when two lines intersect at a point, and one line is horizontal, while the other line is vertical, they form a right angle.
Example: Building Design
In building design, architects often use right angles to create a stable and secure structure. For example, when designing a building, architects may use two intersecting lines with a 90-degree angle to create a corner of the building. This ensures that the building is stable and secure, with a strong foundation.
3. Congruent Angles

Congruent angles are angles that have the same measure. When two lines intersect, and the resulting angles are congruent, they form a right angle. For example, when two lines intersect at a point, and the resulting angles are both 45 degrees, they form a right angle.
Example: Geometry
In geometry, congruent angles are used to prove that two triangles are congruent. When two triangles have congruent angles, they are said to be congruent. For example, when two triangles have angles that are congruent, and the resulting angles form a right angle, the triangles are said to be congruent.
4. Complementary Angles

Complementary angles are angles that add up to 90 degrees. When two lines intersect, and the resulting angles are complementary, they form a right angle. For example, when two lines intersect at a point, and one angle is 60 degrees, while the other angle is 30 degrees, they form a right angle.
Example: Trigonometry
In trigonometry, complementary angles are used to calculate the sine, cosine, and tangent of an angle. When two angles are complementary, they form a right angle, which is essential for trigonometric calculations.
5. Supplementary Angles

Supplementary angles are angles that add up to 180 degrees. When two lines intersect, and the resulting angles are supplementary, they form a straight line, which includes a right angle. For example, when two lines intersect at a point, and one angle is 90 degrees, while the other angle is 90 degrees, they form a straight line, which includes a right angle.
Example: Surveying
In surveying, supplementary angles are used to calculate the angle between two lines. When two lines intersect, and the resulting angles are supplementary, they form a straight line, which is essential for surveying calculations.
6. Alternate Interior Angles

Alternate interior angles are angles that are on opposite sides of a transversal line. When two lines intersect, and the resulting angles are alternate interior angles, they form a right angle. For example, when two lines intersect at a point, and a transversal line intersects the two lines, the resulting angles are alternate interior angles, which form a right angle.
Example: Geometry
In geometry, alternate interior angles are used to prove that two lines are parallel. When two lines intersect, and the resulting angles are alternate interior angles, they form a right angle, which is essential for proving that two lines are parallel.
We hope this article has provided you with a comprehensive understanding of the six ways two lines can form a right angle. Whether you're a student, teacher, or professional, understanding right angles is essential for various mathematical and real-world applications.
What is a right angle?
+A right angle is an angle whose measure is exactly 90 degrees. It is formed when two lines intersect, and the resulting angle is equal to 90 degrees.
How do perpendicular lines form a right angle?
+Perpendicular lines are lines that intersect at a right angle, forming a 90-degree angle.
What are supplementary angles?
+Supplementary angles are angles that add up to 180 degrees. When two lines intersect, and the resulting angles are supplementary, they form a straight line, which includes a right angle.