5 Ways To Master Transformation Form Calculator

Quick Links :

Transformation form calculators are an essential tool for professionals and students in various fields, including mathematics, physics, engineering, and computer science. Mastering these calculators can greatly enhance one's problem-solving skills, accuracy, and efficiency. In this article, we will explore five ways to master transformation form calculators, highlighting their benefits, working mechanisms, and practical applications.

Understanding Transformation Form Calculators

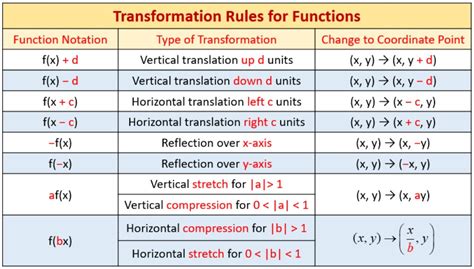
Transformation form calculators are specialized software or calculators designed to perform mathematical transformations, such as converting between different coordinate systems, transforming functions, and calculating derivatives. These calculators are essential for solving complex problems in various fields, including physics, engineering, and computer science.

Types of Transformation Form Calculators

There are several types of transformation form calculators, including:

• Coordinate transformation calculators
• Function transformation calculators
• Derivative calculators
• Integral calculators
• Matrix transformation calculators

Each type of calculator has its unique features, capabilities, and applications.

1. Familiarize Yourself with the Calculator's Interface

To master a transformation form calculator, it's essential to familiarize yourself with its interface. Take some time to explore the calculator's layout, menus, and buttons. Understand how to input data, select functions, and navigate through the calculator's features.

• Read the user manual or online documentation to understand the calculator's capabilities and limitations.
• Practice using the calculator with simple problems to get a feel for its interface and functionality.
• Explore the calculator's built-in functions, such as trigonometric functions, logarithmic functions, and exponential functions.

Benefits of Familiarizing Yourself with the Calculator's Interface

• Improved efficiency: By understanding the calculator's interface, you can quickly and easily access the functions and features you need.
• Reduced errors: Familiarity with the calculator's interface can help you avoid errors caused by incorrect input or navigation.
• Enhanced problem-solving skills: Knowing the calculator's capabilities and limitations can help you choose the best approach to solving complex problems.

2. Practice with Simple Problems

Practicing with simple problems is an excellent way to build your skills and confidence with a transformation form calculator. Start with basic problems, such as converting between different coordinate systems or transforming simple functions.

• Use online resources, such as tutorials, videos, and practice problems, to help you get started.
• Practice regularly to build your skills and muscle memory.
• Gradually increase the complexity of the problems as you become more comfortable with the calculator.

Benefits of Practicing with Simple Problems

• Improved accuracy: Practicing with simple problems can help you develop your accuracy and attention to detail.
• Enhanced problem-solving skills: Simple problems can help you build your problem-solving skills and develop your ability to think critically.
• Increased confidence: Mastering simple problems can give you the confidence to tackle more complex problems.

3. Learn Advanced Techniques and Functions

Once you're comfortable with the calculator's interface and simple problems, it's time to learn advanced techniques and functions. Explore the calculator's built-in functions, such as derivatives, integrals, and matrix operations.

• Read the user manual or online documentation to understand the calculator's advanced features and functions.
• Practice using the calculator with complex problems to build your skills and confidence.
• Explore online resources, such as tutorials, videos, and forums, to learn from experts and other users.

Benefits of Learning Advanced Techniques and Functions

• Improved efficiency: Mastering advanced techniques and functions can help you solve complex problems more quickly and efficiently.
• Enhanced problem-solving skills: Advanced techniques and functions can help you develop your problem-solving skills and think critically.
• Increased versatility: Learning advanced techniques and functions can help you apply the calculator to a wide range of problems and applications.

4. Use the Calculator to Explore Real-World Applications

Transformation form calculators have numerous real-world applications, including physics, engineering, computer science, and mathematics. Use the calculator to explore these applications and develop your problem-solving skills.

• Research real-world problems and applications, such as projectile motion, electrical circuits, and computer graphics.
• Use the calculator to model and solve these problems, exploring the relationships between variables and functions.
• Explore online resources, such as tutorials, videos, and forums, to learn from experts and other users.

Benefits of Using the Calculator to Explore Real-World Applications

• Improved problem-solving skills: Exploring real-world applications can help you develop your problem-solving skills and think critically.
• Enhanced understanding: Using the calculator to model and solve real-world problems can help you develop a deeper understanding of the underlying mathematics and physics.
• Increased motivation: Exploring real-world applications can make mathematics and science more engaging and motivating.

5. Stay Up-to-Date with New Features and Updates

Transformation form calculators are constantly evolving, with new features and updates being released regularly. Stay up-to-date with these developments to ensure you're getting the most out of your calculator.

• Check the manufacturer's website for updates and new features.
• Explore online forums and communities to learn from other users and experts.
• Practice using new features and functions to build your skills and confidence.

Benefits of Staying Up-to-Date with New Features and Updates

• Improved efficiency: New features and updates can help you solve problems more quickly and efficiently.
• Enhanced problem-solving skills: New features and updates can provide new tools and techniques to help you develop your problem-solving skills.
• Increased versatility: Staying up-to-date with new features and updates can help you apply the calculator to a wide range of problems and applications.

Mastering a transformation form calculator takes time and practice, but the benefits are well worth the effort. By following these five tips, you can improve your problem-solving skills, efficiency, and accuracy, and unlock the full potential of your calculator.