2 Times 3 In Fraction Form Made Easy

Quick Links :

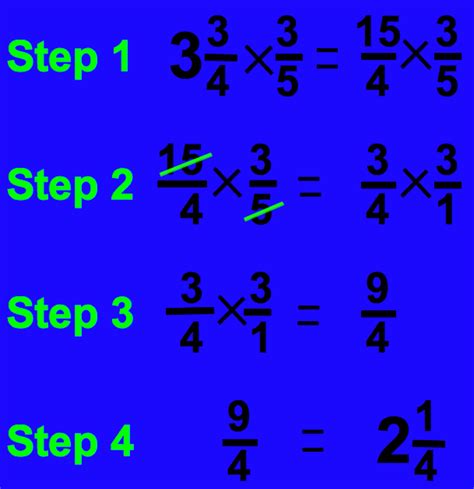
Understanding fractions can be a fundamental concept in mathematics, and it's essential to grasp this idea from a young age. When it comes to solving simple multiplication problems like 2 times 3 in fraction form, it can be a bit tricky. However, with the right approach, it can be made easy.

In everyday life, we often come across situations where we need to multiply fractions. For instance, if you have 2/3 of a pizza and your friend has 3/4 of another pizza, how can you find the total amount of pizza you both have? This is where multiplying fractions comes into play.

To make things clear, let's dive into the basics of multiplying fractions.

Understanding Fractions

A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into.

Fraction Basics

Before we move on to multiplying fractions, let's quickly review some fraction basics:

• A fraction can be proper (numerator < denominator), improper (numerator > denominator), or mixed (a combination of a whole number and a fraction).
• To compare fractions, we need to have the same denominator. We can achieve this by finding the least common multiple (LCM) of the denominators.
• To add or subtract fractions, we need to have the same denominator.

Multiplying Fractions

Multiplying fractions is relatively straightforward. To multiply two fractions, we simply multiply the numerators together to get the new numerator and multiply the denominators together to get the new denominator.

The Multiplication Rule

The rule for multiplying fractions is:

• Multiply the numerators: numerator1 × numerator2
• Multiply the denominators: denominator1 × denominator2
• Write the product as a fraction: (numerator1 × numerator2) / (denominator1 × denominator2)

2 Times 3 in Fraction Form

Now, let's solve the problem of 2 times 3 in fraction form. We can represent 2 as 2/1 and 3 as 3/1.

• Multiply the numerators: 2 × 3 = 6
• Multiply the denominators: 1 × 1 = 1
• Write the product as a fraction: 6/1

So, 2 times 3 in fraction form is 6/1, which can be simplified to 6.

Real-World Applications

Multiplying fractions has many real-world applications. For instance:

• Cooking: When following a recipe, you may need to multiply fractions to scale up or down the ingredients.
• Finance: When calculating interest rates or investment returns, you may need to multiply fractions.
• Science: When measuring quantities in science experiments, you may need to multiply fractions.

Common Mistakes to Avoid

When multiplying fractions, it's essential to avoid common mistakes. Here are a few to watch out for:

• Forgetting to multiply the denominators
• Forgetting to simplify the fraction
• Misplacing the decimal point when converting to decimal form

Tips and Tricks

Here are some tips and tricks to help you master multiplying fractions:

• Practice, practice, practice: The more you practice, the more comfortable you'll become with multiplying fractions.
• Use visual aids: Visual aids like diagrams and charts can help you understand the concept of multiplying fractions.
• Break it down: Break down complex multiplication problems into simpler steps.

Conclusion

Multiplying fractions can seem daunting at first, but with practice and patience, it can become second nature. By following the simple rule of multiplying numerators and denominators, you can solve complex multiplication problems with ease.

We hope this article has helped you understand the concept of multiplying fractions, particularly 2 times 3 in fraction form. With this knowledge, you'll be able to tackle more complex math problems and develop a deeper appreciation for mathematics.

If you have any questions or comments, please feel free to share them below.