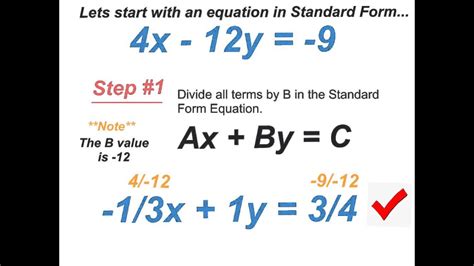
Converting equations from slope-intercept form to standard form can be a daunting task for many students. However, with the right approach and a clear understanding of the concept, it can be accomplished easily. In this article, we will explore the steps involved in converting equations from slope-intercept form to standard form and provide examples to illustrate the process.

The slope-intercept form of an equation is given by y = mx + b, where m represents the slope and b is the y-intercept. On the other hand, the standard form of an equation is given by Ax + By = C, where A, B, and C are integers and A is non-negative.
Why Convert Equations to Standard Form?
Converting equations to standard form can be useful in various mathematical operations, such as solving systems of equations, graphing linear equations, and finding the x and y intercepts. Standard form equations are also easier to compare and analyze, making it a more convenient form for many mathematical applications.
Step-by-Step Conversion Process
Converting an equation from slope-intercept form to standard form involves a few simple steps:
- Multiply both sides of the equation by -1 to eliminate the negative sign in front of the x term.
- Move the constant term to the right-hand side of the equation by subtracting it from both sides.
- Factor out the coefficient of the x term from the left-hand side of the equation.
- Rewrite the equation in standard form by rearranging the terms.
Let's consider an example to illustrate this process:
Example: Convert the equation y = 2x - 3 to standard form.

Solution:
Step 1: Multiply both sides of the equation by -1 to eliminate the negative sign in front of the x term.
- y = 2x - 3
- -y = -2x + 3
Step 2: Move the constant term to the right-hand side of the equation by subtracting it from both sides.
- -y = -2x + 3
- -y - 3 = -2x
Step 3: Factor out the coefficient of the x term from the left-hand side of the equation.
- -y - 3 = -2x
- -2x + y + 3 = 0
Step 4: Rewrite the equation in standard form by rearranging the terms.
- -2x + y + 3 = 0
- 2x - y - 3 = 0
Therefore, the equation y = 2x - 3 can be converted to standard form as 2x - y - 3 = 0.
Common Mistakes to Avoid
When converting equations from slope-intercept form to standard form, there are a few common mistakes to avoid:
- Failing to eliminate the negative sign in front of the x term by multiplying both sides of the equation by -1.
- Forgetting to move the constant term to the right-hand side of the equation.
- Neglecting to factor out the coefficient of the x term from the left-hand side of the equation.
- Failing to rearrange the terms to obtain the standard form of the equation.
Tips and Tricks
Here are a few tips and tricks to help you convert equations from slope-intercept form to standard form easily:
- Always multiply both sides of the equation by -1 to eliminate the negative sign in front of the x term.
- Use the distributive property to factor out the coefficient of the x term from the left-hand side of the equation.
- Rearrange the terms to obtain the standard form of the equation.
- Check your work by plugging in a few values of x to ensure that the equation is true.
By following these steps and tips, you can easily convert equations from slope-intercept form to standard form and simplify your mathematical operations.

In conclusion, converting equations from slope-intercept form to standard form is a simple process that involves a few steps and requires attention to detail. By avoiding common mistakes and using the tips and tricks provided in this article, you can easily convert equations and simplify your mathematical operations.
Take the Next Step
Now that you have learned how to convert equations from slope-intercept form to standard form, it's time to practice and apply your knowledge. Try converting a few equations on your own and check your work to ensure that you are obtaining the correct results.
If you have any questions or need further clarification, please don't hesitate to ask. You can also share your experiences and tips for converting equations in the comments section below.
What is the main difference between slope-intercept form and standard form?
+The main difference between slope-intercept form and standard form is the arrangement of the terms. Slope-intercept form is given by y = mx + b, while standard form is given by Ax + By = C.
Why is it important to convert equations to standard form?
+Converting equations to standard form can be useful in various mathematical operations, such as solving systems of equations, graphing linear equations, and finding the x and y intercepts.
What are some common mistakes to avoid when converting equations to standard form?
+Some common mistakes to avoid when converting equations to standard form include failing to eliminate the negative sign in front of the x term, forgetting to move the constant term to the right-hand side of the equation, neglecting to factor out the coefficient of the x term, and failing to rearrange the terms to obtain the standard form of the equation.