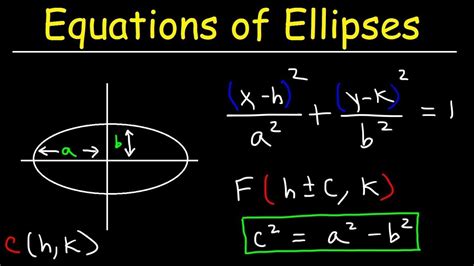
Mastering the standard form equation of an ellipse is a crucial skill for anyone studying mathematics, particularly algebra and geometry. An ellipse is a fundamental concept in mathematics, and understanding its equation is essential for solving problems related to ellipses. In this article, we will explore five ways to master the standard form equation of an ellipse.

Understanding the Standard Form Equation of an Ellipse
The standard form equation of an ellipse is given by:
(x^2/a^2) + (y^2/b^2) = 1
where (a, 0) and (0, b) are the x- and y-intercepts of the ellipse, respectively.
To master this equation, it's essential to understand the properties of an ellipse and how the equation is derived.
Way 1: Visualize the Ellipse
Visualizing the ellipse is a great way to understand its properties and how the equation is derived. Imagine an ellipse with its major axis along the x-axis and its minor axis along the y-axis. The equation of the ellipse can be thought of as a ratio of the distances from the center of the ellipse to the x- and y-intercepts.

Key Takeaway: Visualizing the ellipse helps to understand the relationship between the x- and y-intercepts and the equation of the ellipse.
Way 2: Derive the Equation from the Definition of an Ellipse
Another way to master the standard form equation of an ellipse is to derive it from the definition of an ellipse. An ellipse is defined as the set of all points (x, y) in a plane such that the sum of the distances from (x, y) to two fixed points (called foci) is constant.
Using this definition, we can derive the equation of the ellipse as follows:
Let (x, y) be a point on the ellipse, and let (c, 0) and (-c, 0) be the foci. Then, the sum of the distances from (x, y) to the foci is given by:
√((x-c)^2 + y^2) + √((x+c)^2 + y^2) = 2a
where a is the semi-major axis of the ellipse.
Simplifying this equation, we get:
(x^2/a^2) + (y^2/b^2) = 1

Key Takeaway: Deriving the equation of the ellipse from its definition helps to understand the underlying mathematics and properties of the ellipse.
Way 3: Use Graphing Techniques
Graphing is a powerful technique for visualizing and understanding the equation of an ellipse. By graphing the equation, we can see the shape of the ellipse and how it changes as the values of a and b change.
To graph the equation of an ellipse, we can use a graphing calculator or software. Simply enter the equation and adjust the values of a and b to see how the ellipse changes.

Key Takeaway: Graphing the equation of an ellipse helps to visualize and understand the properties of the ellipse.
Way 4: Practice, Practice, Practice
Practice is essential for mastering the standard form equation of an ellipse. By working through examples and exercises, we can develop a deeper understanding of the equation and how to apply it to solve problems.
Here are a few examples to get you started:
Example 1: Find the equation of the ellipse with x-intercepts (2, 0) and (−2, 0) and y-intercepts (0, 3) and (0, −3).
Example 2: Find the length of the major axis of the ellipse with equation (x^2/4) + (y^2/9) = 1.
Example 3: Find the foci of the ellipse with equation (x^2/16) + (y^2/25) = 1.

Key Takeaway: Practice is essential for mastering the standard form equation of an ellipse.
Way 5: Use Real-World Applications
Finally, using real-world applications is a great way to master the standard form equation of an ellipse. By seeing how the equation is used in real-world contexts, we can develop a deeper understanding of its importance and relevance.
Here are a few examples of real-world applications of the equation of an ellipse:
Example 1: Satellite orbits. The orbit of a satellite around the Earth is an ellipse, with the Earth at one of the foci.
Example 2: Mirrors. Elliptical mirrors are used in optics to focus light and images.
Example 3: Architecture. Elliptical shapes are used in architecture to design buildings and bridges.

Key Takeaway: Using real-world applications helps to see the relevance and importance of the equation of an ellipse.
Final Thoughts
Mastering the standard form equation of an ellipse requires a combination of visualization, derivation, graphing, practice, and real-world applications. By following these five ways, you can develop a deep understanding of the equation and its properties, and apply it to solve problems in mathematics and real-world contexts.
We encourage you to share your thoughts and experiences with mastering the standard form equation of an ellipse in the comments below.
What is the standard form equation of an ellipse?
+The standard form equation of an ellipse is (x^2/a^2) + (y^2/b^2) = 1.
How do I derive the equation of an ellipse from its definition?
+Deriving the equation of an ellipse from its definition involves using the definition of an ellipse as the set of all points (x, y) in a plane such that the sum of the distances from (x, y) to two fixed points (called foci) is constant.
What are some real-world applications of the equation of an ellipse?
+Some real-world applications of the equation of an ellipse include satellite orbits, mirrors, and architecture.