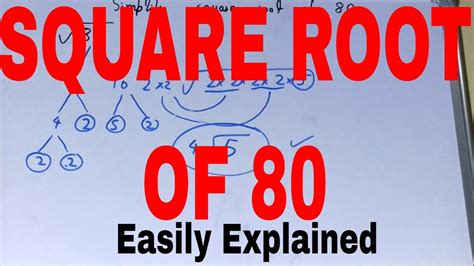
Simplify The Square Root Of 80 In 2 Easy Steps

Quick Links :

Simplify The Square Root Of 80 In 2 Easy Steps

The square root of 80 can seem like a daunting task, but it's actually quite simple once you understand the process. In this article, we'll break down the steps to simplify the square root of 80 in just two easy steps.

Why Simplify the Square Root of 80?

Before we dive into the steps, let's quickly discuss why simplifying the square root of 80 is important. In mathematics, simplifying radical expressions helps to make calculations easier and more manageable. It's especially crucial in algebra, geometry, and other mathematical disciplines. By simplifying the square root of 80, we can make it easier to work with and understand mathematical concepts.

Step 1: Factor 80 into Prime Factors

The first step in simplifying the square root of 80 is to factor 80 into its prime factors. Prime factorization involves breaking down a number into its simplest building blocks, which are prime numbers. To factor 80, we need to find the prime numbers that multiply together to give 80.

The prime factorization of 80 is:

80 = 2 × 2 × 2 × 2 × 5

Or, in a more compact form:

80 = 2^4 × 5

What's the Significance of Prime Factorization?

Prime factorization is essential in simplifying radical expressions because it helps us identify perfect squares. In the case of 80, we can see that it's not a perfect square because it doesn't have an even power of each prime factor.

Step 2: Simplify the Square Root of 80

Now that we have the prime factorization of 80, we can simplify the square root of 80. To do this, we'll use the following rule:

√(ab) = √a × √b

where a and b are prime factors.

Using this rule, we can simplify the square root of 80 as follows:

√80 = √(2^4 × 5) = √(2^2 × 2^2 × 5) = √(4 × 4 × 5) = 4√5

And that's it! We've successfully simplified the square root of 80 in just two easy steps.

What Does the Simplified Answer Mean?

The simplified answer, 4√5, means that the square root of 80 is equal to 4 times the square root of 5. This makes it easier to work with and understand mathematical concepts that involve the square root of 80.

Additional Tips and Tricks

Here are some additional tips and tricks to help you simplify radical expressions:

• Always factor the radicand (the number inside the square root) into its prime factors.
• Look for perfect squares within the radicand.
• Use the rule √(ab) = √a × √b to simplify radical expressions.

By following these tips and tricks, you'll become a pro at simplifying radical expressions in no time!

Common Mistakes to Avoid

When simplifying radical expressions, it's essential to avoid common mistakes. Here are some mistakes to watch out for:

• Forgetting to factor the radicand into its prime factors.
• Not identifying perfect squares within the radicand.
• Using the wrong rule to simplify radical expressions.

By avoiding these common mistakes, you'll ensure that your simplifications are accurate and correct.

Conclusion

In conclusion, simplifying the square root of 80 is a straightforward process that involves factoring the radicand into its prime factors and using the rule √(ab) = √a × √b. By following these steps, you'll be able to simplify radical expressions with ease. Remember to avoid common mistakes and use additional tips and tricks to help you become a pro at simplifying radical expressions.

We hope this article has been helpful in simplifying the square root of 80. If you have any questions or comments, please leave them below!