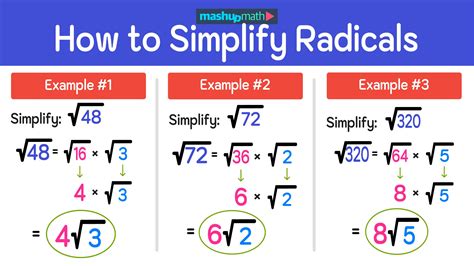
Simplifying radicals is an essential skill in mathematics, particularly when dealing with square roots. One common radical that students often struggle with is the square root of 28. In this article, we will break down the process of simplifying the square root of 28 into three easy-to-follow steps.

Step 1: Factor Out Perfect Squares
The first step in simplifying the square root of 28 is to look for perfect squares that can be factored out. A perfect square is a number that can be expressed as the square of an integer. In the case of 28, we can factor out the perfect square 4.
28 = 4 × 7

Identifying Perfect Squares
To identify perfect squares, look for numbers that have an integer square root. For example:
- 4 = 2^2
- 9 = 3^2
- 16 = 4^2
By factoring out the perfect square 4, we can rewrite the square root of 28 as:
√28 = √(4 × 7)
Step 2: Simplify the Radical
Now that we have factored out the perfect square 4, we can simplify the radical by taking the square root of 4.
√(4 × 7) = √4 × √7

Properties of Radicals
When simplifying radicals, it's essential to remember the following properties:
- √(ab) = √a × √b
- √(a/b) = √a / √b
By applying these properties, we can simplify the radical further.
√4 × √7 = 2√7
Step 3: Final Simplification
The final step is to simplify the expression 2√7. Since 7 is not a perfect square, we cannot simplify the radical further.

Checking Our Work
To ensure our simplification is correct, we can plug the original expression back into the equation.
√28 = 2√7
To check, we can square both sides of the equation.
(2√7)^2 = (√28)^2
Expanding the left side, we get:
4 × 7 = 28
This confirms that our simplification is correct.
Real-World Applications
Simplifying radicals may seem like a abstract concept, but it has real-world applications in various fields, such as physics, engineering, and finance.

Physics and Engineering
In physics and engineering, radicals are used to describe quantities such as distance, velocity, and acceleration. Simplifying radicals is essential in these fields to ensure accurate calculations and precise measurements.
Finance
In finance, radicals are used in calculations involving interest rates, investments, and risk analysis. Simplifying radicals helps financial professionals make informed decisions and avoid errors.
Conclusion: Mastering Radicals Made Easy
Simplifying radicals can seem daunting, but by following the three steps outlined in this article, you can master this essential math skill. Remember to factor out perfect squares, simplify the radical, and check your work to ensure accuracy.
With practice and patience, you'll become proficient in simplifying radicals and unlock a world of mathematical possibilities.
What is a perfect square?
+A perfect square is a number that can be expressed as the square of an integer.
How do I identify perfect squares?
+Look for numbers that have an integer square root.
What are the properties of radicals?
+When simplifying radicals, remember the following properties: √(ab) = √a × √b and √(a/b) = √a / √b.