5 Ways To Express Square Root In Exponent Form

Quick Links :

Expressing square roots in exponent form is a fundamental concept in mathematics, particularly in algebra and calculus. The square root of a number can be rewritten using exponents, providing a more compact and versatile representation. This concept is crucial for simplifying complex expressions, solving equations, and manipulating mathematical formulas. In this article, we will explore five ways to express square roots in exponent form, along with practical examples and explanations.

What is the Square Root in Exponent Form?

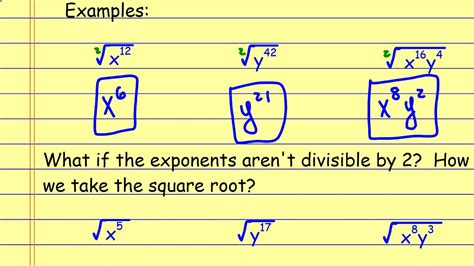
The square root of a number x can be expressed as x^(1/2), where the exponent 1/2 represents the square root operation. This notation is equivalent to the radical symbol √x. For instance, √16 can be rewritten as 16^(1/2), which simplifies to 4.

Benefits of Exponent Form

Expressing square roots in exponent form offers several benefits, including:

• Simplified calculations: Exponent form can make calculations more straightforward, especially when dealing with complex expressions.
• Easier manipulation: Exponent form allows for more flexibility when manipulating mathematical formulas and equations.
• Compact representation: Exponent form provides a more concise representation of square roots, making it easier to read and write.

Method 1: Simple Square Roots

The simplest way to express a square root in exponent form is to replace the radical symbol with the exponent 1/2. For example:

√x = x^(1/2) √16 = 16^(1/2) = 4

This method applies to any square root expression, making it a fundamental concept in mathematics.

Example

Express √25 in exponent form.

√25 = 25^(1/2) = 5

Method 2: Negative Square Roots

When dealing with negative square roots, the exponent form remains the same. The negative sign is absorbed into the exponent, resulting in a positive exponent with a negative base. For example:

-√x = -(x^(1/2)) -√16 = -(16^(1/2)) = -4

This method ensures that the negative sign is properly accounted for in the exponent form.

Example

Express -√9 in exponent form.

-√9 = -(9^(1/2)) = -3

Method 3: Fractional Exponents

Fractional exponents can be used to express square roots with a non-integer exponent. This method involves multiplying the exponent by 1/2 to obtain the square root. For example:

x^(1/2) = √x x^(3/2) = √(x^3)

This method provides a more general approach to expressing square roots in exponent form.

Example

Express x^(3/2) in radical form.

x^(3/2) = √(x^3)

Method 4: Rational Exponents

Rational exponents involve a ratio of integers in the exponent. This method can be used to express square roots with a rational exponent. For example:

x^(m/n) = (x^m)^(1/n) x^(2/3) = (√(x^2))^3

This method provides a more comprehensive approach to expressing square roots in exponent form.

Example

Express x^(2/3) in radical form.

x^(2/3) = (√(x^2))^3

Method 5: Irrational Exponents

Irrational exponents involve a non-repeating, non-terminating decimal in the exponent. This method can be used to express square roots with an irrational exponent. For example:

x^(π) = x^(3.14159...) x^(e) = x^(2.71828...)

This method provides a more advanced approach to expressing square roots in exponent form.

Example

Express x^(π) in radical form.

x^(π) = x^(3.14159...)

In conclusion, expressing square roots in exponent form is a powerful tool for simplifying complex expressions and manipulating mathematical formulas. By mastering the five methods outlined in this article, you can improve your mathematical skills and tackle even the most challenging problems with confidence.