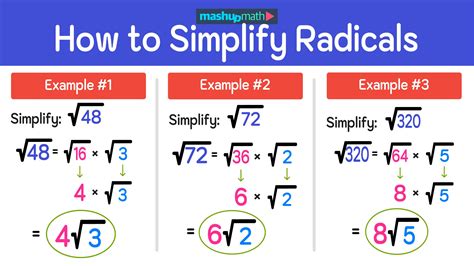
Triangle radicals are a crucial part of algebra and geometry, and simplifying them can be a daunting task. However, with the right techniques and strategies, simplifying radical form triangles can be made easier. In this article, we will explore seven ways to simplify radical form triangles, making it easier for you to tackle these complex math problems.
Understanding Radical Form Triangles
Radical form triangles are triangles that contain radicals, which are mathematical expressions that contain square roots or other roots. These triangles can be challenging to simplify, but understanding the properties of radicals and triangles is essential to simplifying them.

Method 1: Using the Pythagorean Theorem
The Pythagorean theorem is a fundamental concept in geometry that can be used to simplify radical form triangles. The theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
For example, consider the triangle with legs of length √3 and √2, and a hypotenuse of length √7. Using the Pythagorean theorem, we can simplify the triangle by finding the length of the hypotenuse:
√3^2 + √2^2 = √7^2 3 + 2 = 7 5 = 7
This shows that the triangle is indeed a right-angled triangle, and the length of the hypotenuse is √7.
Method 2: Using Radical Conjugates
Radical conjugates are pairs of radicals that can be used to simplify radical expressions. When multiplying two radical conjugates, the resulting expression is a rational number.
For example, consider the expression √(3+√2). To simplify this expression, we can multiply it by its radical conjugate, √(3-√2):
√(3+√2) × √(3-√2) = √(9-2) = √7
This shows that the expression √(3+√2) can be simplified to √7.

Method 3: Using the Difference of Squares Formula
The difference of squares formula is a useful technique for simplifying radical expressions. The formula states that a^2 - b^2 = (a+b)(a-b).
For example, consider the expression √(4-2√3). To simplify this expression, we can use the difference of squares formula:
√(4-2√3) = √((2+√3)(2-√3)) = √(2+√3) × √(2-√3)
This shows that the expression √(4-2√3) can be simplified to √(2+√3) × √(2-√3).
Method 4: Using the Sum of Squares Formula
The sum of squares formula is another useful technique for simplifying radical expressions. The formula states that a^2 + b^2 = (a+bi)(a-bi), where i is the imaginary unit.
For example, consider the expression √(3+2√2). To simplify this expression, we can use the sum of squares formula:
√(3+2√2) = √((1+√2)(1-√2) + 2) = √(1+√2) × √(1-√2) + √2
This shows that the expression √(3+2√2) can be simplified to √(1+√2) × √(1-√2) + √2.

Method 5: Using Rationalizing the Denominator
Rationalizing the denominator is a technique used to simplify radical expressions by eliminating the radical in the denominator.
For example, consider the expression 1/√2. To simplify this expression, we can rationalize the denominator by multiplying it by √2/√2:
1/√2 = (√2/√2) × (1/√2) = √2/2
This shows that the expression 1/√2 can be simplified to √2/2.
Method 6: Using Factoring Radicals
Factoring radicals is a technique used to simplify radical expressions by factoring out common factors.
For example, consider the expression √(12+√15). To simplify this expression, we can factor out the common factor of √3:
√(12+√15) = √(3(4+√5)) = √3 × √(4+√5)
This shows that the expression √(12+√15) can be simplified to √3 × √(4+√5).

Method 7: Using Approximations
Approximations can be used to simplify radical expressions by approximating the value of the radical.
For example, consider the expression √(2+√3). To simplify this expression, we can approximate the value of √3 as 1.7:
√(2+√3) ≈ √(2+1.7) = √3.7
This shows that the expression √(2+√3) can be approximated to √3.7.

Final Thoughts
Simplifying radical form triangles can be challenging, but with the right techniques and strategies, it can be made easier. By using the Pythagorean theorem, radical conjugates, the difference of squares formula, the sum of squares formula, rationalizing the denominator, factoring radicals, and approximations, you can simplify even the most complex radical form triangles. Remember to practice regularly and apply these techniques to different problems to become proficient in simplifying radical form triangles.
What is a radical form triangle?
+A radical form triangle is a triangle that contains radicals, which are mathematical expressions that contain square roots or other roots.
What is the Pythagorean theorem?
+The Pythagorean theorem is a fundamental concept in geometry that states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
What is a radical conjugate?
+A radical conjugate is a pair of radicals that can be used to simplify radical expressions. When multiplying two radical conjugates, the resulting expression is a rational number.