Simplify Radicals In 1 Easy Step: 45s Simplest Form

Quick Links :

Radicals can be intimidating, especially when they're part of a complex mathematical expression. However, simplifying radicals can be a straightforward process, and with the right approach, you can break down even the most complicated radicals into their simplest form. In this article, we'll explore a simple step-by-step method for simplifying radicals, which can be applied to any radical expression.

Why Simplify Radicals?

Before we dive into the simplification process, let's quickly discuss why simplifying radicals is important. Simplifying radicals helps to:

• Reduce the complexity of mathematical expressions
• Make calculations easier and more manageable
• Improve the overall understanding of mathematical concepts
• Enhance problem-solving skills

The Simplest Form Method

To simplify radicals, we'll use the following easy step:

Step 1: Find the Largest Perfect Square Factor

The key to simplifying radicals is to find the largest perfect square factor of the radicand (the number inside the radical). A perfect square is a number that can be expressed as the square of an integer, such as 4, 9, 16, or 25.

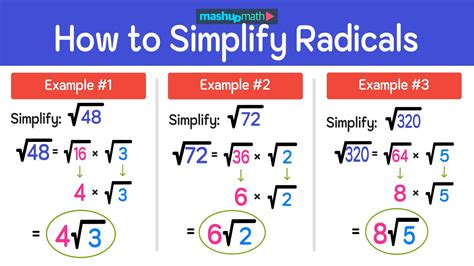
For example, let's simplify the radical √48.

Breaking Down the Radicand

To simplify √48, we need to find the largest perfect square factor of 48. The factors of 48 are 1, 2, 3, 4, 6, 8, 12, 16, 24, and 48. Among these factors, 16 is the largest perfect square (4² = 16).

Simplifying the Radical

Now that we've found the largest perfect square factor, we can simplify the radical:

√48 = √(16 × 3)

= √16 × √3

= 4√3

And that's it! By finding the largest perfect square factor, we've simplified the radical √48 into its simplest form, 4√3.

Applying the Simplest Form Method

Let's apply this method to a few more examples:

• √75 = √(25 × 3) = √25 × √3 = 5√3
• √200 = √(100 × 2) = √100 × √2 = 10√2
• √125 = √(25 × 5) = √25 × √5 = 5√5

As you can see, this method is easy to apply and can be used to simplify any radical expression.

Tips and Tricks

Here are some additional tips to keep in mind when simplifying radicals:

• Always look for the largest perfect square factor.
• Use the properties of radicals, such as √(ab) = √a × √b.
• Simplify radicals as soon as possible to avoid working with complex expressions.

Real-World Applications

Simplifying radicals has numerous real-world applications, including:

• Physics: Radicals are used to describe quantities such as distance, velocity, and acceleration.
• Engineering: Radicals are used to calculate stresses, strains, and loads on structures.
• Computer Science: Radicals are used in algorithms for tasks such as image processing and machine learning.

Conclusion

Simplifying radicals is a straightforward process that can be mastered with practice. By following the simple step of finding the largest perfect square factor, you can break down even the most complicated radicals into their simplest form. Remember to apply this method consistently, and you'll become proficient in simplifying radicals in no time.

What's Next?

Now that you've learned how to simplify radicals, you can move on to more advanced topics, such as:

• Solving radical equations
• Graphing radical functions
• Applying radicals to real-world problems

Share your thoughts and feedback in the comments below! Have you struggled with simplifying radicals in the past? How do you think this method can help you in your mathematical journey?