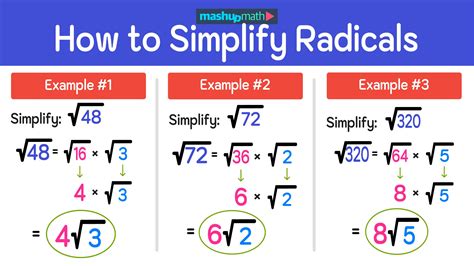
Simplifying radicals is a fundamental concept in mathematics, and it's essential to understand the process to solve various math problems. In this article, we'll break down the steps to simplify radicals, using the example of the square root of 20.
Understanding Radicals

Radicals are mathematical expressions that contain a root, such as a square root or cube root. They are used to represent numbers that cannot be expressed as simple fractions. The square root of a number, denoted by the symbol √, is a value that, when multiplied by itself, gives the original number. For example, √16 = 4, because 4 multiplied by 4 equals 16.
Why Simplify Radicals?
Simplifying radicals is essential to make mathematical expressions more manageable and easier to work with. Simplified radicals can be added, subtracted, multiplied, and divided, just like any other numbers. This simplification process helps to:
- Reduce complexity: Simplifying radicals makes it easier to understand and work with mathematical expressions.
- Improve accuracy: Simplified radicals reduce the risk of errors when performing mathematical operations.
- Enhance problem-solving: Simplifying radicals is a crucial step in solving various math problems, including algebra, geometry, and calculus.
The 3 Easy Steps to Simplify Radicals

Now that we understand the importance of simplifying radicals, let's dive into the 3 easy steps to simplify the square root of 20.
Step 1: Factor the Number Inside the Radical
The first step in simplifying the square root of 20 is to factor the number inside the radical. We can break down 20 into its prime factors: 20 = 2 × 2 × 5.
Step 2: Identify Perfect Squares
The next step is to identify perfect squares within the factored expression. In this case, we have 2 × 2, which is a perfect square (2^2 = 4).
Step 3: Simplify the Radical
Now, we can simplify the radical by taking the square root of the perfect square (2^2) and multiplying it by the remaining factor (5). This gives us:
√20 = √(2^2 × 5) = 2√5
And that's it! We have simplified the square root of 20 in just 3 easy steps.
Practical Applications of Simplifying Radicals

Simplifying radicals has numerous practical applications in various fields, including:
- Algebra: Simplifying radicals is essential for solving algebraic equations and inequalities.
- Geometry: Radicals are used to calculate distances, lengths, and areas in geometry.
- Calculus: Simplifying radicals is crucial for solving optimization problems and finding maximum and minimum values.
Real-World Examples
- Architecture: Architects use simplified radicals to calculate the height of buildings and the length of shadows.
- Physics: Physicists use radicals to calculate distances, velocities, and accelerations in motion.
- Engineering: Engineers use simplified radicals to design and optimize systems, such as bridges and electronic circuits.
Common Mistakes to Avoid When Simplifying Radicals

When simplifying radicals, it's essential to avoid common mistakes that can lead to incorrect results. Here are some common mistakes to watch out for:
- Not factoring the number inside the radical correctly
- Not identifying perfect squares correctly
- Not simplifying the radical correctly
By avoiding these common mistakes, you can ensure accurate results when simplifying radicals.
Conclusion: Mastering the Art of Simplifying Radicals

Simplifying radicals is a fundamental concept in mathematics that requires practice and patience to master. By following the 3 easy steps outlined in this article, you can simplify radicals with confidence. Remember to factor the number inside the radical, identify perfect squares, and simplify the radical correctly. With practice, you'll become proficient in simplifying radicals and tackle even the most complex math problems with ease.
We encourage you to share your thoughts and feedback on this article. Have you struggled with simplifying radicals in the past? What techniques have you found helpful? Share your experiences and tips in the comments below.
What is a radical in mathematics?
+A radical is a mathematical expression that contains a root, such as a square root or cube root.
Why is simplifying radicals important?
+Simplifying radicals makes mathematical expressions more manageable and easier to work with. It also reduces complexity, improves accuracy, and enhances problem-solving.
What are some common mistakes to avoid when simplifying radicals?
+Common mistakes to avoid include not factoring the number inside the radical correctly, not identifying perfect squares correctly, and not simplifying the radical correctly.