Simplifying radicals is an essential concept in mathematics, particularly in algebra and geometry. It involves expressing a radical expression in its simplest form, making it easier to work with and understand. In this article, we will explore the world of radicals, their importance, and provide easy steps to simplify them to their simplest form.
Radicals are mathematical expressions that contain a square root, cube root, or higher-order root. They are used to represent numbers that cannot be expressed as simple fractions or integers. Radicals are commonly encountered in various mathematical operations, such as solving equations, finding distances, and calculating areas. However, working with radicals can be challenging, especially when they are not in their simplest form. Simplifying radicals is crucial to avoid errors and make mathematical calculations more manageable.
The importance of simplifying radicals cannot be overstated. When radicals are in their simplest form, they are easier to work with, and mathematical operations become more straightforward. Simplifying radicals also helps to avoid errors and ensures that calculations are accurate. In this article, we will provide a step-by-step guide on how to simplify radicals, making it easier for you to work with these mathematical expressions.
Understanding Radicals

Before we dive into the steps of simplifying radicals, it is essential to understand the basics of radicals. A radical expression consists of a root symbol (√), a radicand (the number inside the root symbol), and an index (the number outside the root symbol). The index indicates the type of root, such as square root, cube root, or higher-order root.
Radicand and Index
The radicand is the number inside the root symbol, while the index is the number outside the root symbol. The index indicates the type of root, such as square root (index 2), cube root (index 3), or higher-order root.
Easy Steps to Simplify Radicals

Simplifying radicals involves a series of steps that help to express the radical expression in its simplest form. Here are the easy steps to simplify radicals:
Step 1: Factor the Radicand
The first step in simplifying radicals is to factor the radicand. Factoring the radicand helps to identify any perfect squares, cubes, or higher-order powers that can be simplified.
Step 2: Identify Perfect Squares, Cubes, or Higher-Order Powers
Identify any perfect squares, cubes, or higher-order powers within the radicand. Perfect squares are numbers that can be expressed as a product of two identical integers, such as 16 = 4 × 4. Perfect cubes are numbers that can be expressed as a product of three identical integers, such as 27 = 3 × 3 × 3.
Step 3: Simplify the Radical Expression
Simplify the radical expression by extracting the perfect squares, cubes, or higher-order powers from the radicand. For example, √16 = √(4 × 4) = 4, and ∛27 = ∛(3 × 3 × 3) = 3.
Step 4: Rationalize the Denominator (If Necessary)
If the radical expression has a denominator with a radical, rationalize the denominator by multiplying both the numerator and denominator by the conjugate of the radical.
Examples of Simplifying Radicals

Here are some examples of simplifying radicals:
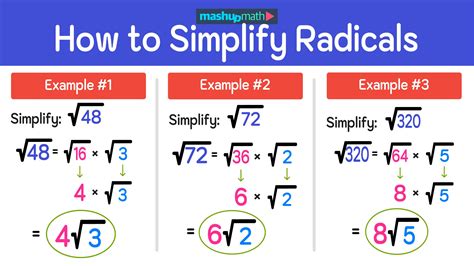
Example 1: Simplify √16
√16 = √(4 × 4) = 4
Example 2: Simplify ∛27
∛27 = ∛(3 × 3 × 3) = 3
Example 3: Simplify √(2 × 2 × 5)
√(2 × 2 × 5) = √(4 × 5) = √4 × √5 = 2√5
Practical Applications of Simplifying Radicals

Simplifying radicals has numerous practical applications in various fields, including mathematics, physics, engineering, and computer science. Here are some examples of practical applications of simplifying radicals:
Distance Calculations
Simplifying radicals is essential in distance calculations, particularly in physics and engineering. For example, calculating the distance between two points in a coordinate system involves simplifying radicals.
Area and Volume Calculations
Simplifying radicals is also crucial in area and volume calculations. For example, calculating the area of a circle or the volume of a sphere involves simplifying radicals.
Equation Solving
Simplifying radicals is essential in solving equations, particularly in algebra and calculus. For example, solving quadratic equations involves simplifying radicals.
Conclusion

Simplifying radicals is an essential concept in mathematics, and it is crucial to understand the steps involved in simplifying radicals. By following the easy steps outlined in this article, you can simplify radicals with ease and make mathematical calculations more manageable. Remember, simplifying radicals is essential in various practical applications, including distance calculations, area and volume calculations, and equation solving.
We hope this article has provided you with a comprehensive understanding of simplifying radicals. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and comments below, and don't forget to share this article with your friends and colleagues.
What is the purpose of simplifying radicals?
+The purpose of simplifying radicals is to express the radical expression in its simplest form, making it easier to work with and understand.
What are the steps involved in simplifying radicals?
+The steps involved in simplifying radicals include factoring the radicand, identifying perfect squares, cubes, or higher-order powers, simplifying the radical expression, and rationalizing the denominator (if necessary).
What are some practical applications of simplifying radicals?
+Some practical applications of simplifying radicals include distance calculations, area and volume calculations, and equation solving.