Simplify Radicals With Ease Using Our Simple Calculator

Quick Links :

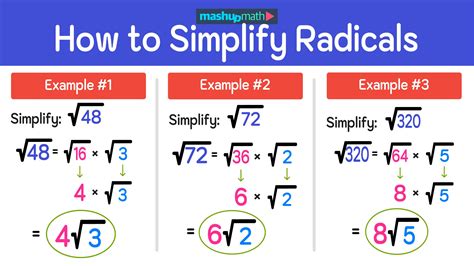
Simplifying radicals can be a daunting task, especially for those who are new to algebra. However, with the right tools and techniques, it can be a breeze. In this article, we will explore the concept of radicals, their importance in mathematics, and provide a step-by-step guide on how to simplify them using our simple calculator.

Radicals are mathematical expressions that contain a square root or other roots, such as cube roots or fourth roots. They are used to represent numbers that cannot be expressed as simple fractions or decimals. Radicals are commonly used in algebra, geometry, and calculus to solve equations and manipulate expressions.

Why Simplify Radicals?

Simplifying radicals is essential in mathematics because it allows us to work with expressions more efficiently. When radicals are simplified, they can be added, subtracted, multiplied, and divided more easily. Simplifying radicals also helps to reveal the underlying structure of mathematical expressions, making it easier to solve equations and manipulate formulas.

How to Simplify Radicals Using Our Calculator

Our simple calculator is designed to make simplifying radicals a breeze. Here's a step-by-step guide on how to use it:

1. Enter the radical expression: Simply type in the radical expression you want to simplify into the calculator.
2. Choose the operation: Select the operation you want to perform, such as simplifying a square root or cube root.
3. Click calculate: Click the calculate button, and the calculator will simplify the radical expression for you.

Understanding the Basics of Radical Simplification

Before we dive into the calculator, it's essential to understand the basics of radical simplification. Here are some key concepts to keep in mind:

• Square roots: Square roots are the most common type of radical. They are denoted by the symbol √ and represent a number that, when multiplied by itself, gives a specified value.
• Cube roots: Cube roots are denoted by the symbol ∛ and represent a number that, when multiplied by itself twice, gives a specified value.
• Fourth roots: Fourth roots are denoted by the symbol √[4] and represent a number that, when multiplied by itself three times, gives a specified value.

Step-by-Step Guide to Simplifying Radicals

Here's a step-by-step guide to simplifying radicals:

1. Factor out perfect squares: Look for perfect squares within the radical expression and factor them out.
2. Simplify the radical: Simplify the radical by finding the square root, cube root, or fourth root of the number inside the radical.
3. Rationalize the denominator: If the radical is in the denominator, rationalize it by multiplying the numerator and denominator by the conjugate of the denominator.

Common Mistakes to Avoid When Simplifying Radicals

When simplifying radicals, there are several common mistakes to avoid:

• Not factoring out perfect squares: Failing to factor out perfect squares can lead to incorrect simplification.
• Not rationalizing the denominator: Failing to rationalize the denominator can lead to incorrect simplification.
• Not simplifying the radical: Failing to simplify the radical can lead to incorrect simplification.

Conclusion

Simplifying radicals can be a challenging task, but with the right tools and techniques, it can be made easier. Our simple calculator is designed to make simplifying radicals a breeze, and by following the step-by-step guide outlined in this article, you can master the art of radical simplification.