5 Ways To Simplify Radical Form Exponents

Quick Links :

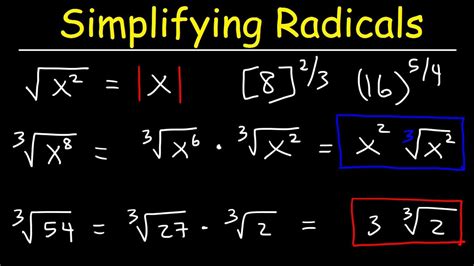
Simplifying radical form exponents can be a daunting task for many students, but with the right strategies, it can become a manageable and even enjoyable process. In this article, we will explore five ways to simplify radical form exponents, making it easier for you to tackle even the most complex problems.

Understanding Radical Form Exponents

Before we dive into the simplification methods, it's essential to understand what radical form exponents are. A radical form exponent is a mathematical expression that consists of a root (such as a square root or cube root) raised to a power. For example, √(x^3) is a radical form exponent. These types of expressions can be challenging to simplify, but with the right techniques, you can master them.

Method 1: Simplifying Using the Properties of Exponents

One of the most effective ways to simplify radical form exponents is to use the properties of exponents. The product rule, power rule, and quotient rule can be applied to radical form exponents to simplify them. For example, using the product rule, we can simplify √(x^3) × √(x^4) by combining the exponents: √(x^3 × x^4) = √(x^7).

Here are some examples of simplifying radical form exponents using the properties of exponents:

• √(x^3) × √(x^4) = √(x^7)
• (√(x^2))^3 = x^3
• √(x^6) / √(x^2) = √(x^4)

Benefits of Using Exponent Properties

Using exponent properties to simplify radical form exponents has several benefits. It allows you to:

• Simplify complex expressions quickly and efficiently
• Combine multiple radical form exponents into a single expression
• Reduce the complexity of the expression, making it easier to work with

Method 2: Simplifying Using the Laws of Exponents

Another effective way to simplify radical form exponents is to use the laws of exponents. The laws of exponents state that when multiplying exponential expressions with the same base, we add the exponents. When dividing exponential expressions with the same base, we subtract the exponents. Using these laws, we can simplify radical form exponents by adding or subtracting the exponents.

Here are some examples of simplifying radical form exponents using the laws of exponents:

• √(x^3) × √(x^4) = √(x^3+4) = √(x^7)
• (√(x^2))^3 = x^(2×3) = x^6
• √(x^6) / √(x^2) = √(x^6-2) = √(x^4)

Benefits of Using Laws of Exponents

Using the laws of exponents to simplify radical form exponents has several benefits. It allows you to:

• Simplify complex expressions by adding or subtracting exponents
• Combine multiple radical form exponents into a single expression
• Reduce the complexity of the expression, making it easier to work with

Method 3: Simplifying Using Rational Exponents

Rational exponents can also be used to simplify radical form exponents. Rational exponents are exponents that are expressed as fractions, such as 1/2 or 3/4. By converting radical form exponents to rational exponents, we can simplify them using the laws of exponents.

Here are some examples of simplifying radical form exponents using rational exponents:

• √(x^3) = x^(3/2)
• (√(x^2))^3 = x^(2/3) × x^(3/1) = x^3
• √(x^6) / √(x^2) = x^(6/2) / x^(2/2) = x^2

Benefits of Using Rational Exponents

Using rational exponents to simplify radical form exponents has several benefits. It allows you to:

• Convert radical form exponents to a more manageable form
• Simplify complex expressions using the laws of exponents
• Reduce the complexity of the expression, making it easier to work with

Method 4: Simplifying Using Radical Conjugates

Radical conjugates can also be used to simplify radical form exponents. Radical conjugates are pairs of expressions that have the same terms but opposite signs. By multiplying radical form exponents by their conjugates, we can eliminate the radical sign and simplify the expression.

Here are some examples of simplifying radical form exponents using radical conjugates:

• √(x^3) × √(x^3) = x^3
• (√(x^2))^3 = x^2 × x^2 × x^2 = x^6
• √(x^6) / √(x^2) = √(x^6) × √(x^2) / x^2 = x^2

Benefits of Using Radical Conjugates

Using radical conjugates to simplify radical form exponents has several benefits. It allows you to:

• Eliminate the radical sign and simplify the expression
• Multiply radical form exponents by their conjugates to eliminate the radical sign
• Reduce the complexity of the expression, making it easier to work with

Method 5: Simplifying Using Factoring

Factoring can also be used to simplify radical form exponents. By factoring out perfect squares or cubes, we can simplify radical form exponents and eliminate the radical sign.

Here are some examples of simplifying radical form exponents using factoring:

• √(x^4) = √(x^2 × x^2) = x^2
• (√(x^2))^3 = x^2 × x^2 × x^2 = x^6
• √(x^6) / √(x^2) = √(x^4) = x^2

Benefits of Using Factoring

Using factoring to simplify radical form exponents has several benefits. It allows you to:

• Factor out perfect squares or cubes to simplify the expression
• Eliminate the radical sign and simplify the expression
• Reduce the complexity of the expression, making it easier to work with

We hope this article has helped you understand the different methods for simplifying radical form exponents. By using these methods, you can simplify even the most complex expressions and make them easier to work with. Remember to practice regularly to become more comfortable with these methods.