5 Ways To Master Point Slope Form With Practice

Quick Links :

Learning to master point-slope form is an essential skill for any student of mathematics, particularly in algebra and geometry. Point-slope form is a way of expressing the equation of a line, and it is used extensively in mathematics, physics, engineering, and many other fields. By mastering point-slope form, you can become proficient in solving problems involving linear equations, graphing lines, and analyzing functions.

In this article, we will explore five ways to master point-slope form with practice, including explanations, examples, and exercises to help you become proficient in using this powerful mathematical tool.

Understanding Point-Slope Form

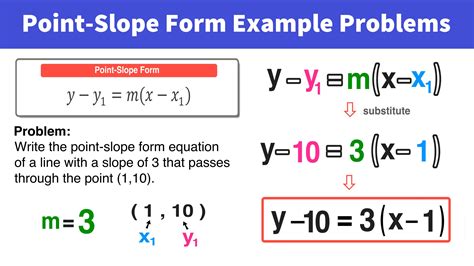
Point-slope form is a way of expressing the equation of a line in the form y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope of the line. The slope of a line is a measure of how steep it is, and it is calculated by dividing the change in y by the change in x.

To master point-slope form, you need to understand the concept of slope and how to use it to write the equation of a line. You also need to practice writing equations in point-slope form and solving problems involving linear equations.

Why Master Point-Slope Form?

Mastering point-slope form has several benefits, including:

• Improved problem-solving skills: By mastering point-slope form, you can become proficient in solving problems involving linear equations, which are used extensively in mathematics, physics, engineering, and many other fields.
• Better understanding of graphing: Point-slope form is used to graph lines, which is an essential skill in mathematics and science. By mastering point-slope form, you can become proficient in graphing lines and analyzing functions.
• Enhanced critical thinking: Mastering point-slope form requires critical thinking and problem-solving skills, which can help you develop a deeper understanding of mathematical concepts and improve your analytical skills.

5 Ways to Master Point-Slope Form with Practice

Here are five ways to master point-slope form with practice:

1. Start with the Basics

To master point-slope form, you need to start with the basics. Make sure you understand the concept of slope and how to calculate it. Practice writing equations in point-slope form using simple examples, such as finding the equation of a line given two points.

• Example: Find the equation of the line passing through the points (2, 3) and (4, 5).
• Solution: First, calculate the slope using the formula m = (y2 - y1) / (x2 - x1). Then, use the point-slope form to write the equation of the line.

2. Practice Writing Equations in Point-Slope Form

Practice writing equations in point-slope form using different examples. Start with simple examples, such as finding the equation of a line given two points, and gradually move on to more complex examples, such as finding the equation of a line given a point and a slope.

• Example: Find the equation of the line passing through the point (3, 4) with a slope of 2.
• Solution: Use the point-slope form to write the equation of the line.

3. Solve Problems Involving Linear Equations

Practice solving problems involving linear equations, such as finding the x-intercept or the y-intercept of a line. Use point-slope form to write the equation of the line and then solve for the intercepts.

• Example: Find the x-intercept of the line passing through the points (2, 3) and (4, 5).
• Solution: First, write the equation of the line in point-slope form. Then, solve for the x-intercept by setting y = 0 and solving for x.

4. Use Graphing to Visualize Point-Slope Form

Use graphing to visualize point-slope form. Graph lines using point-slope form and analyze the graph to understand the relationship between the slope and the y-intercept.

• Example: Graph the line passing through the point (3, 4) with a slope of 2.
• Solution: Use point-slope form to write the equation of the line. Then, graph the line and analyze the graph to understand the relationship between the slope and the y-intercept.

5. Practice with Real-World Applications

Practice using point-slope form with real-world applications, such as physics, engineering, or economics. Use point-slope form to model real-world problems and solve for unknowns.

• Example: A car travels from point A to point B at an average speed of 60 miles per hour. If the distance between the two points is 120 miles, how long does it take for the car to travel from point A to point B?
• Solution: Use point-slope form to model the problem and solve for the unknown.

Conclusion

Mastering point-slope form takes practice and dedication, but it is an essential skill for any student of mathematics. By following these five ways to master point-slope form with practice, you can become proficient in using this powerful mathematical tool and improve your problem-solving skills.

We encourage you to try the examples and exercises provided in this article to practice using point-slope form. With practice and dedication, you can master point-slope form and become proficient in solving problems involving linear equations.