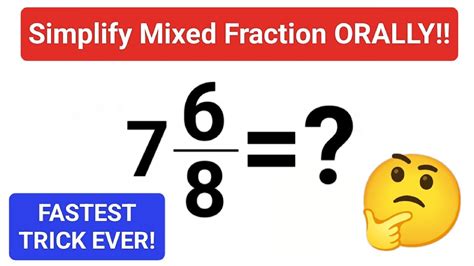Simplifying Mixed Fractions Made Easy

Quick Links :

Simplifying Mixed Fractions: Understanding the Basics

Mixed fractions can be a bit tricky to work with, especially when it comes to simplifying them. However, with a solid understanding of the basics and some practice, simplifying mixed fractions can become a breeze. In this article, we'll take a closer look at what mixed fractions are, how to simplify them, and provide some practical examples to help you master this skill.

At its core, a mixed fraction is a combination of a whole number and a proper fraction. For example, 3 1/2 is a mixed fraction, where 3 is the whole number and 1/2 is the proper fraction. Mixed fractions can be used to represent a wide range of real-world quantities, from measurements to recipe ingredients. However, when working with mixed fractions, it's often necessary to simplify them to make calculations easier.

Why Simplify Mixed Fractions?

So, why is it important to simplify mixed fractions? There are several reasons why simplifying mixed fractions is a crucial skill to master:

• Simplifying mixed fractions makes it easier to perform calculations, such as addition and subtraction.
• Simplified mixed fractions can be more easily compared and ordered.
• Simplifying mixed fractions is often necessary when working with fractions in real-world applications, such as cooking and construction.

How to Simplify Mixed Fractions

Now that we've covered the basics, let's dive into the steps for simplifying mixed fractions.

Step 1: Convert the Mixed Fraction to an Improper Fraction

To simplify a mixed fraction, we need to first convert it to an improper fraction. To do this, multiply the whole number by the denominator and add the numerator. This will give us the new numerator. The denominator remains the same.

For example, let's say we want to simplify the mixed fraction 3 1/2. We can convert it to an improper fraction by multiplying the whole number (3) by the denominator (2) and adding the numerator (1). This gives us:

(3 x 2) + 1 = 7

So, the improper fraction is 7/2.

Step 2: Simplify the Improper Fraction

Now that we have the improper fraction, we can simplify it by dividing the numerator by the greatest common divisor (GCD) of the numerator and denominator.

For example, let's say we want to simplify the improper fraction 7/2. The GCD of 7 and 2 is 1, so we can't simplify it any further.

Step 3: Convert the Simplified Improper Fraction Back to a Mixed Fraction (Optional)

If we want to express the simplified fraction as a mixed fraction, we can convert it back by dividing the numerator by the denominator and writing the remainder as the new numerator.

For example, let's say we want to convert the simplified improper fraction 7/2 back to a mixed fraction. We can divide the numerator (7) by the denominator (2) and write the remainder as the new numerator:

7 ÷ 2 = 3 with a remainder of 1

So, the mixed fraction is 3 1/2.

Practical Examples

Let's take a look at some practical examples of simplifying mixed fractions:

• Simplify the mixed fraction 2 3/4.

Convert the mixed fraction to an improper fraction: (2 x 4) + 3 = 11

The improper fraction is 11/4.

Simplify the improper fraction: The GCD of 11 and 4 is 1, so we can't simplify it any further.

• Simplify the mixed fraction 1 2/3.

Convert the mixed fraction to an improper fraction: (1 x 3) + 2 = 5

The improper fraction is 5/3.

Simplify the improper fraction: The GCD of 5 and 3 is 1, so we can't simplify it any further.

Tips and Tricks for Simplifying Mixed Fractions

Here are some tips and tricks for simplifying mixed fractions:

• Make sure to convert the mixed fraction to an improper fraction first.
• Use the greatest common divisor (GCD) to simplify the improper fraction.
• If the numerator is larger than the denominator, you may need to convert the improper fraction back to a mixed fraction.
• Practice, practice, practice! Simplifying mixed fractions takes time and practice to master.

Common Mistakes to Avoid

Here are some common mistakes to avoid when simplifying mixed fractions:

• Forgetting to convert the mixed fraction to an improper fraction first.
• Using the wrong GCD to simplify the improper fraction.
• Not converting the improper fraction back to a mixed fraction when necessary.
• Not practicing enough to master the skill.

Conclusion

Simplifying mixed fractions is a crucial skill to master, especially when working with fractions in real-world applications. By following the steps outlined in this article and practicing regularly, you can become proficient in simplifying mixed fractions.

Whether you're a student, teacher, or simply someone who wants to improve their math skills, we hope this article has been helpful in your journey to master simplifying mixed fractions.

Now it's your turn! Share your thoughts, questions, and experiences with simplifying mixed fractions in the comments below. What tips and tricks do you have to share? Let's continue the conversation!

Like and share this article with your friends and family who may benefit from learning about simplifying mixed fractions.

Stay tuned for more math-related articles and tutorials!

FAQ Section