Mastering Slope-Intercept Form: Ixl Answer Key Insights

Quick Links :

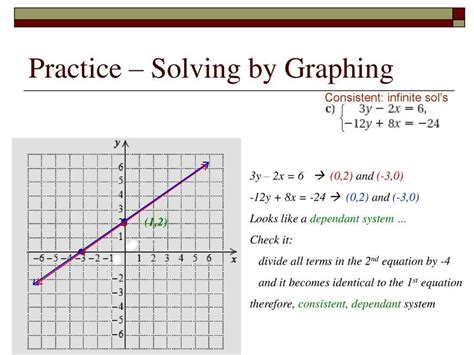
Slope-intercept form is a fundamental concept in algebra and mathematics, allowing students to understand and work with linear equations in a more intuitive and meaningful way. The slope-intercept form, denoted as y = mx + b, where m represents the slope and b represents the y-intercept, is a powerful tool for solving equations, graphing lines, and modeling real-world problems. However, mastering this concept can be a daunting task for many students, especially when it comes to applying it to complex problems.

Understanding the Basics of Slope-Intercept Form

At its core, the slope-intercept form is a way of representing linear equations in a way that highlights the relationship between the slope and the y-intercept. The slope, or m, represents the rate of change of the line, while the y-intercept, or b, represents the point at which the line crosses the y-axis. By understanding the relationship between these two components, students can gain a deeper insight into the behavior and characteristics of linear equations.

Breaking Down the Slope-Intercept Form

The slope-intercept form can be broken down into its component parts:

• Slope (m): The slope represents the rate of change of the line, indicating how steep the line is and whether it rises or falls as x increases.
• Y-intercept (b): The y-intercept represents the point at which the line crosses the y-axis, indicating the starting point of the line.

IXL Answer Key Insights: Tips and Strategies for Mastering Slope-Intercept Form

IXL, a popular online learning platform, offers a range of resources and tools to help students master slope-intercept form. The IXL answer key provides detailed explanations and solutions to help students understand and apply the concept. Here are some tips and strategies for mastering slope-intercept form using the IXL answer key:

• Start with the basics: Begin by reviewing the fundamental concepts of slope-intercept form, including the slope and y-intercept.
• Practice, practice, practice: Use the IXL answer key to practice solving problems and applying the slope-intercept form to different types of equations.
• Focus on graphing: Graphing is a critical component of understanding slope-intercept form. Use the IXL answer key to practice graphing lines and identifying key features, such as the slope and y-intercept.
• Use real-world examples: Apply the slope-intercept form to real-world problems and scenarios to gain a deeper understanding of its practical applications.

Common Mistakes to Avoid

When working with slope-intercept form, there are several common mistakes to avoid:

• Incorrectly identifying the slope and y-intercept: Make sure to carefully identify the slope and y-intercept, as these values are critical to solving problems.
• Confusing slope-intercept form with other forms of linear equations: Be careful not to confuse slope-intercept form with other forms of linear equations, such as point-slope form or standard form.
• Failing to graph the line correctly: Graphing is a critical component of understanding slope-intercept form. Make sure to graph the line correctly, including identifying key features such as the slope and y-intercept.

Advanced Applications of Slope-Intercept Form

Once you have mastered the basics of slope-intercept form, you can begin to explore more advanced applications. Some examples include:

• Linear regression: Use slope-intercept form to model real-world data and make predictions about future outcomes.
• Optimization problems: Use slope-intercept form to solve optimization problems, such as finding the maximum or minimum value of a function.
• System of equations: Use slope-intercept form to solve systems of equations, where multiple equations intersect.

Real-World Examples of Slope-Intercept Form

Slope-intercept form has a wide range of real-world applications, including:

• Physics and engineering: Slope-intercept form is used to model the motion of objects, including the trajectory of projectiles and the motion of cars.
• Economics: Slope-intercept form is used to model economic systems, including supply and demand curves.
• Computer science: Slope-intercept form is used to model algorithms and optimize code.

Now that you have gained a deeper understanding of slope-intercept form and its applications, it's time to put your knowledge to the test. Try working through some practice problems using the IXL answer key, and explore real-world examples of slope-intercept form in action.