Write A Polynomial In Standard Form Easily

Quick Links :

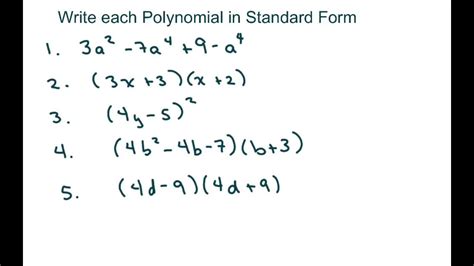
Polynomials are a fundamental concept in algebra, and writing them in standard form is a crucial skill for any math enthusiast. In this article, we will explore the world of polynomials and provide a step-by-step guide on how to write a polynomial in standard form with ease.

What is a Polynomial?

A polynomial is an algebraic expression consisting of variables and coefficients combined using only addition, subtraction, and multiplication. Polynomials can have one or more terms, and each term is a product of a coefficient and one or more variables raised to a non-negative power.

Key Characteristics of Polynomials

Before we dive into writing polynomials in standard form, let's review some key characteristics:

• Polynomials can have one or more terms.
• Each term is a product of a coefficient and one or more variables.
• Variables are raised to non-negative powers.
• Polynomials can have a constant term.

What is Standard Form?

Standard form is a way of writing polynomials in a specific format, making it easier to work with and compare them. In standard form, a polynomial is written with the terms arranged in descending order of exponents.

Benefits of Standard Form

Writing polynomials in standard form has several benefits:

• Easier comparison: Standard form makes it easier to compare polynomials and determine if they are equivalent.
• Simplified calculations: Standard form simplifies calculations, such as addition and subtraction of polynomials.
• Improved understanding: Standard form helps to improve understanding of polynomial properties and behavior.

Step-by-Step Guide to Writing a Polynomial in Standard Form

Now that we have covered the basics, let's move on to the step-by-step guide:

1. Combine like terms: Combine any like terms in the polynomial. Like terms are terms that have the same variable(s) raised to the same power.
2. Arrange terms in descending order: Arrange the terms in descending order of exponents. This means the term with the highest exponent should come first.
3. Write the polynomial: Write the polynomial with the terms arranged in descending order, using a positive coefficient for the first term.

Example: Writing a Polynomial in Standard Form

Suppose we have the polynomial:

2x^3 + 5x^2 - 3x + 1

To write this polynomial in standard form, we follow the steps:

1. Combine like terms: There are no like terms in this polynomial.
2. Arrange terms in descending order: The terms are already in descending order of exponents.
3. Write the polynomial: The polynomial is already written in standard form.

The polynomial 2x^3 + 5x^2 - 3x + 1 is in standard form.

Tips and Tricks for Writing Polynomials in Standard Form

Here are some tips and tricks to help you write polynomials in standard form:

• Always combine like terms before arranging the terms in descending order.
• Use a positive coefficient for the first term.
• Make sure to write the polynomial in descending order of exponents.

Common Mistakes to Avoid

Here are some common mistakes to avoid when writing polynomials in standard form:

• Forgetting to combine like terms.
• Arranging terms in ascending order instead of descending order.
• Using a negative coefficient for the first term.

Conclusion

Writing polynomials in standard form is a crucial skill in algebra. By following the step-by-step guide and tips and tricks, you can easily write polynomials in standard form. Remember to combine like terms, arrange terms in descending order, and use a positive coefficient for the first term. With practice and patience, you will become proficient in writing polynomials in standard form.