5 Ways To Rewrite Quadratic Functions In Standard Form

Quick Links :

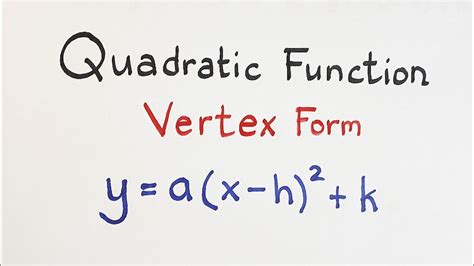
Quadratic functions are a fundamental concept in algebra, and rewriting them in standard form is an essential skill for any math student. The standard form of a quadratic function is ax^2 + bx + c, where a, b, and c are constants, and x is the variable. In this article, we will explore five different ways to rewrite quadratic functions in standard form, along with examples and explanations.

The importance of rewriting quadratic functions in standard form cannot be overstated. By converting a quadratic function to standard form, you can easily identify the vertex, axis of symmetry, and roots of the parabola, making it easier to analyze and solve problems. Moreover, standard form is a universal language that allows mathematicians and scientists to communicate and compare quadratic functions from different contexts.

Method 1: Factoring Out the Greatest Common Factor (GCF)

Factoring out the greatest common factor (GCF) is a simple and effective method to rewrite quadratic functions in standard form. The GCF is the largest expression that divides both terms of the quadratic function without leaving a remainder. By factoring out the GCF, you can rewrite the quadratic function as a product of two binomials.

For example, consider the quadratic function 6x^2 + 12x + 18. The GCF of the terms is 6, so we can factor out 6 from each term:

6x^2 + 12x + 18 = 6(x^2 + 2x + 3)

This is the standard form of the quadratic function.

Steps to Factor Out the GCF

• Identify the GCF of the terms
• Factor out the GCF from each term
• Simplify the expression

Method 2: Using the Quadratic Formula

The quadratic formula is a powerful tool to rewrite quadratic functions in standard form. The quadratic formula is:

x = (-b ± √(b^2 - 4ac)) / 2a

By using the quadratic formula, you can rewrite the quadratic function as:

ax^2 + bx + c = a(x - (-b ± √(b^2 - 4ac)) / 2a)^2

For example, consider the quadratic function x^2 + 5x + 6. By using the quadratic formula, we can rewrite it as:

x^2 + 5x + 6 = (x + 2)^2 - 1

This is the standard form of the quadratic function.

Steps to Use the Quadratic Formula

• Write down the quadratic formula
• Plug in the values of a, b, and c
• Simplify the expression

Method 3: Completing the Square

Completing the square is a method to rewrite quadratic functions in standard form by adding and subtracting a constant term. By completing the square, you can rewrite the quadratic function as:

ax^2 + bx + c = a(x + b/2a)^2 - (b^2 - 4ac) / 4a

For example, consider the quadratic function x^2 + 4x + 4. By completing the square, we can rewrite it as:

x^2 + 4x + 4 = (x + 2)^2

This is the standard form of the quadratic function.

Steps to Complete the Square

• Write down the quadratic function
• Add and subtract a constant term to make the expression a perfect square
• Simplify the expression

Method 4: Using Graphical Methods

Graphical methods involve plotting the quadratic function on a graph and identifying the vertex, axis of symmetry, and roots. By using graphical methods, you can rewrite the quadratic function in standard form.

For example, consider the quadratic function x^2 + 2x + 1. By plotting the graph, we can identify the vertex as (-1, 0) and the axis of symmetry as x = -1. Therefore, we can rewrite the quadratic function as:

x^2 + 2x + 1 = (x + 1)^2

This is the standard form of the quadratic function.

Steps to Use Graphical Methods

• Plot the quadratic function on a graph
• Identify the vertex, axis of symmetry, and roots
• Rewrite the quadratic function in standard form

Method 5: Using Algebraic Manipulation

Algebraic manipulation involves rearranging the terms of the quadratic function to rewrite it in standard form. By using algebraic manipulation, you can rewrite the quadratic function as:

ax^2 + bx + c = a(x^2 + bx/a + c/a)

For example, consider the quadratic function 2x^2 + 4x + 2. By using algebraic manipulation, we can rewrite it as:

2x^2 + 4x + 2 = 2(x^2 + 2x + 1)

This is the standard form of the quadratic function.

Steps to Use Algebraic Manipulation

• Write down the quadratic function
• Rearrange the terms to rewrite the quadratic function in standard form
• Simplify the expression

In conclusion, rewriting quadratic functions in standard form is an essential skill for any math student. By mastering the five methods outlined in this article, you can easily rewrite quadratic functions in standard form, making it easier to analyze and solve problems.