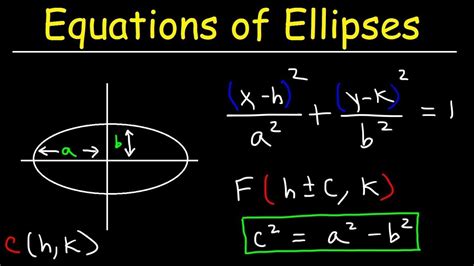
Ellipse equations are a fundamental concept in mathematics, particularly in algebra and geometry. The standard form of an ellipse equation is essential to understand, as it helps in solving various problems and applications involving ellipses.
In this article, we will delve into the world of ellipses and explore the 5 steps to convert an ellipse equation into its standard form. But before we begin, let's understand the importance of ellipse equations and their applications.
Understanding Ellipse Equations

Ellipse equations are used to represent the shape and size of an ellipse in a two-dimensional plane. An ellipse is a closed curve, resulting from the intersection of a cone by a plane cutting at an angle to the plane of the base. The equation of an ellipse helps in determining its major and minor axes, which are crucial in various mathematical and real-world applications.
Applications of Ellipse Equations

Ellipse equations have numerous applications in various fields, including:
- Astronomy: Ellipse equations are used to describe the orbits of planets, comets, and other celestial bodies.
- Engineering: Ellipse equations are used in the design of bridges, tunnels, and other structures.
- Physics: Ellipse equations are used to model the motion of objects in two-dimensional space.
- Computer Graphics: Ellipse equations are used to create smooth curves and shapes in computer graphics.
Step 1: Identify the General Form of an Ellipse Equation

The general form of an ellipse equation is:
Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0
where A, B, C, D, E, and F are constants.
Understanding the Coefficients
- A and C represent the coefficients of the squared terms (x^2 and y^2).
- B represents the coefficient of the cross term (xy).
- D and E represent the coefficients of the linear terms (x and y).
- F represents the constant term.
Step 2: Group the Terms and Factor Out the Greatest Common Factor

Group the terms with the same variable (x or y) and factor out the greatest common factor (GCF).
Example:
Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0
Group the x terms: Ax^2 + Bxy + Dx
Group the y terms: Cy^2 + Ey
Factor out the GCF:
A(x^2 + (B/A)xy + (D/A)x) + C(y^2 + (E/C)y) + F = 0
Step 3: Complete the Square for the x and y Terms

Complete the square for the x and y terms by adding and subtracting the appropriate constants.
Example:
A(x^2 + (B/A)xy + (D/A)x) + C(y^2 + (E/C)y) + F = 0
Complete the square for x: A(x^2 + (B/A)xy + (D/A)x + (B/2A)^2) - A(B/2A)^2
Complete the square for y: C(y^2 + (E/C)y + (E/2C)^2) - C(E/2C)^2
Step 4: Write the Equation in Standard Form

Write the equation in standard form by combining the completed squares and constants.
Example:
A(x + (B/2A))^2 + C(y + (E/2C))^2 = (A(B/2A)^2 + C(E/2C)^2 - F)
Standard form:
((x + (B/2A))^2)/((B/2A)^2) + ((y + (E/2C))^2)/((E/2C)^2) = 1
Step 5: Simplify the Equation (Optional)

Simplify the equation by dividing both sides by the constant term, if necessary.
Example:
((x + (B/2A))^2)/((B/2A)^2) + ((y + (E/2C))^2)/((E/2C)^2) = 1
Simplify:
(x + (B/2A))^2/((B/2A)^2) + (y + (E/2C))^2/((E/2C)^2) = 1
And that's it! By following these 5 steps, you can convert any ellipse equation into its standard form.
What is the standard form of an ellipse equation?
+The standard form of an ellipse equation is ((x - h)^2)/a^2 + ((y - k)^2)/b^2 = 1, where (h,k) is the center of the ellipse, and a and b are the lengths of the semi-major and semi-minor axes, respectively.
What are the applications of ellipse equations?
+Ellipse equations have numerous applications in various fields, including astronomy, engineering, physics, and computer graphics.
How do I simplify an ellipse equation?
+To simplify an ellipse equation, divide both sides by the constant term, if necessary.
We hope you found this article informative and helpful. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences with ellipse equations in the comments below.