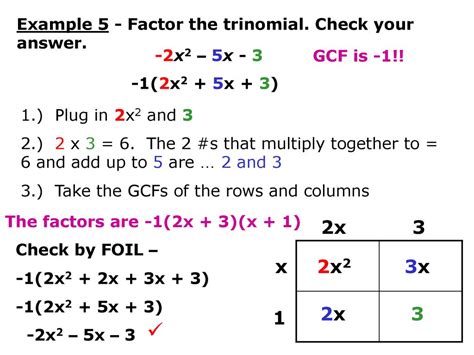
Factoring Trinomials Made Easy: Ax2+Bx+C Answer Key

Quick Links :

Factoring trinomials can be a daunting task for many students, but with the right approach, it can be made easy. In this article, we will explore the concept of factoring trinomials, specifically those of the form ax2 + bx + c, and provide a step-by-step guide on how to solve them.

The Importance of Factoring Trinomials

Factoring trinomials is an essential skill in algebra, as it allows us to solve quadratic equations, simplify expressions, and solve problems in various fields such as physics, engineering, and computer science. By factoring trinomials, we can break down complex expressions into simpler components, making it easier to work with them.

Understanding the Form ax2 + bx + c

A trinomial is a polynomial with three terms. In the case of ax2 + bx + c, we have a quadratic trinomial, where a, b, and c are constants. The coefficient a is the leading coefficient, b is the linear coefficient, and c is the constant term.

To factor a trinomial of this form, we need to find two binomials whose product is equal to the original trinomial. This can be achieved by finding the factors of the constant term c and the leading coefficient a.

Step-by-Step Guide to Factoring Trinomials

Step 1: Check if the Trinomial is Factorable

Before we start factoring, we need to check if the trinomial is factorable. To do this, we need to check if the discriminant b2 - 4ac is a perfect square. If it is, then the trinomial can be factored.

Step 2: Find the Factors of the Constant Term c

Once we have confirmed that the trinomial is factorable, we need to find the factors of the constant term c. This can be done by listing all the possible factors of c and finding the pair that adds up to the linear coefficient b.

Step 3: Write the Factored Form

Once we have found the factors of the constant term c, we can write the factored form of the trinomial. This is done by multiplying the two binomials together to get the original trinomial.

Examples of Factoring Trinomials

Let's consider a few examples of factoring trinomials:

• x2 + 5x + 6
• 2x2 + 7x + 3
• x2 - 4x - 3

In each of these examples, we can follow the steps outlined above to factor the trinomial.

Example 1: x2 + 5x + 6

To factor this trinomial, we need to find the factors of the constant term 6. The factors of 6 are 1, 2, 3, and 6. We can then check which pair of factors adds up to the linear coefficient 5. In this case, the factors are 2 and 3, so we can write the factored form as (x + 2)(x + 3).

Example 2: 2x2 + 7x + 3

To factor this trinomial, we need to find the factors of the constant term 3. The factors of 3 are 1 and 3. We can then check which pair of factors adds up to the linear coefficient 7. In this case, the factors are 1 and 6, so we can write the factored form as (2x + 1)(x + 3).

Example 3: x2 - 4x - 3

To factor this trinomial, we need to find the factors of the constant term -3. The factors of -3 are -1 and 3. We can then check which pair of factors adds up to the linear coefficient -4. In this case, the factors are -1 and -3, so we can write the factored form as (x - 1)(x - 3).

Tips and Tricks for Factoring Trinomials

• Always check if the trinomial is factorable before attempting to factor it.
• Use the factors of the constant term c to find the factored form.
• Check your work by multiplying the two binomials together to get the original trinomial.
• Practice, practice, practice! The more you practice factoring trinomials, the easier it will become.

Common Mistakes to Avoid

• Not checking if the trinomial is factorable before attempting to factor it.
• Not using the correct factors of the constant term c.
• Not checking your work by multiplying the two binomials together.

Conclusion

Factoring trinomials can seem daunting at first, but with practice and patience, it can become second nature. By following the steps outlined above and avoiding common mistakes, you can become a pro at factoring trinomials in no time. Remember to always check if the trinomial is factorable, use the correct factors of the constant term c, and check your work by multiplying the two binomials together.

Final Thoughts

Factoring trinomials is an essential skill in algebra, and with the right approach, it can be made easy. By understanding the form ax2 + bx + c and following the steps outlined above, you can become proficient in factoring trinomials. Remember to practice regularly and avoid common mistakes to become a master of factoring trinomials.

We hope this article has helped you understand the concept of factoring trinomials and has provided you with the tools and techniques needed to become proficient in this area. If you have any questions or comments, please feel free to share them below.