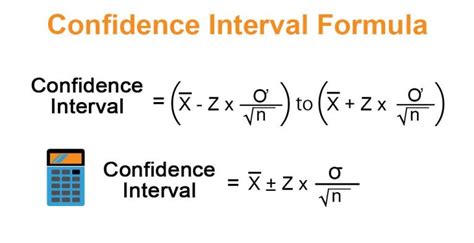
In the realm of statistical analysis, confidence intervals are a crucial tool for expressing the reliability of estimates. These intervals provide a range of values within which a population parameter is likely to lie, giving researchers and analysts a sense of the uncertainty associated with their estimates. However, there are different ways to express confidence intervals, and the choice of method can significantly impact the interpretation of results. In this article, we will explore three common ways to express confidence intervals and discuss their implications.
What are Confidence Intervals?

Before diving into the different ways to express confidence intervals, it's essential to understand what they represent. A confidence interval is a range of values that is likely to contain the true population parameter. The width of the interval is determined by the sample size, the variability of the data, and the desired level of confidence. For example, a 95% confidence interval indicates that there is a 95% probability that the true population parameter lies within the specified range.
Method 1: Margin of Error

One common way to express confidence intervals is through the margin of error. The margin of error is the maximum amount by which the sample estimate may differ from the true population parameter. For example, if a survey reports a 95% confidence interval for the average height of adults with a margin of error of 2 inches, it means that there is a 95% probability that the true average height lies within 2 inches of the sample estimate. The margin of error is a simple and intuitive way to express confidence intervals, but it can be misleading if not interpreted correctly.
Pros and Cons of Margin of Error
Pros:
- Easy to understand and communicate
- Provides a clear sense of the uncertainty associated with the estimate
Cons:
- Can be misleading if the interval is asymmetric or skewed
- Does not provide information about the shape or distribution of the interval
Method 2: Interval Notation

Another way to express confidence intervals is through interval notation. Interval notation represents the confidence interval as a range of values, typically in the format [lower bound, upper bound]. For example, a 95% confidence interval for the average height of adults might be expressed as [67.2, 72.8] inches. Interval notation provides a clear and concise way to express confidence intervals, but it can be less intuitive than the margin of error for non-technical audiences.
Pros and Cons of Interval Notation
Pros:
- Provides a clear and concise representation of the interval
- Easy to compute and analyze
Cons:
- Can be less intuitive for non-technical audiences
- Does not provide information about the uncertainty associated with the estimate
Method 3: Probability Distribution

A more advanced way to express confidence intervals is through probability distributions. A probability distribution represents the likelihood of different values occurring within the interval. For example, a normal distribution might be used to model the confidence interval for the average height of adults. Probability distributions provide a rich and nuanced way to express confidence intervals, but they can be challenging to interpret and communicate to non-technical audiences.
Pros and Cons of Probability Distributions
Pros:
- Provides a rich and nuanced representation of the interval
- Allows for the modeling of complex distributions and relationships
Cons:
- Can be challenging to interpret and communicate to non-technical audiences
- Requires advanced statistical knowledge and expertise
What is the difference between a confidence interval and a probability distribution?
+A confidence interval represents a range of values within which a population parameter is likely to lie, while a probability distribution represents the likelihood of different values occurring within the interval.
How do I choose the right method for expressing confidence intervals?
+The choice of method depends on the audience, the complexity of the data, and the level of precision required. Margin of error is suitable for simple and intuitive communication, interval notation is ideal for technical audiences, and probability distributions are best for advanced statistical analysis.
What is the importance of confidence intervals in statistical analysis?
+Confidence intervals provide a sense of the uncertainty associated with estimates, allowing researchers and analysts to make informed decisions and communicate results effectively.
In conclusion, expressing confidence intervals is a crucial aspect of statistical analysis. The choice of method depends on the audience, the complexity of the data, and the level of precision required. By understanding the different ways to express confidence intervals, researchers and analysts can effectively communicate their results and make informed decisions. We hope this article has provided valuable insights into the world of confidence intervals and encourages you to explore further. Don't hesitate to share your thoughts and questions in the comments section below!