5 Ways To Simplify Expressions With Exponential Form

Quick Links :

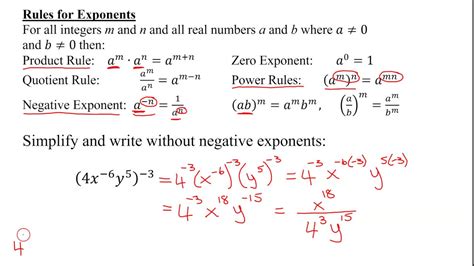
Simplifying expressions with exponential form is an essential skill in mathematics, particularly in algebra and calculus. Exponential expressions can be intimidating, but with the right strategies, you can simplify them with ease. In this article, we will explore five ways to simplify expressions with exponential form, making it easier for you to tackle complex mathematical problems.

Understanding Exponential Form

Before we dive into simplifying exponential expressions, it's essential to understand what exponential form is. Exponential form represents a number as a product of a base and an exponent. The base is the number being multiplied, and the exponent is the number of times the base is multiplied by itself. For example, 2^3 represents 2 multiplied by itself three times, which equals 8.

Method 1: Simplifying Exponential Expressions with the Same Base

When simplifying exponential expressions with the same base, you can combine the exponents by adding or subtracting them. For example, 2^3 × 2^4 can be simplified by adding the exponents: 2^(3+4) = 2^7. This rule applies to division as well: 2^3 ÷ 2^4 can be simplified by subtracting the exponents: 2^(3-4) = 2^(-1).

Examples:

• 3^2 × 3^5 = 3^(2+5) = 3^7
• 4^3 ÷ 4^2 = 4^(3-2) = 4^1
• 2^3 × 2^(-2) = 2^(3-2) = 2^1

Method 2: Simplifying Exponential Expressions with Different Bases

When simplifying exponential expressions with different bases, you cannot combine the exponents. However, you can simplify the expression by evaluating the exponents separately. For example, 2^3 × 3^4 can be simplified by evaluating the exponents: 2^3 = 8 and 3^4 = 81, then multiplying the results: 8 × 81 = 648.

Examples:

• 2^3 × 3^2 = 8 × 9 = 72
• 4^2 × 2^3 = 16 × 8 = 128
• 3^4 × 2^(-2) = 81 × 1/4 = 20.25

Method 3: Simplifying Exponential Expressions with Negative Exponents

When simplifying exponential expressions with negative exponents, you can rewrite the expression with a positive exponent by flipping the base and changing the sign of the exponent. For example, 2^(-3) can be rewritten as 1/(2^3). This rule applies to fractions as well: 3/2^(-2) can be rewritten as 3 × 2^2.

Examples:

• 2^(-3) = 1/(2^3) = 1/8
• 3^(-2) = 1/(3^2) = 1/9
• 4^(-1) = 1/(4^1) = 1/4

Method 4: Simplifying Exponential Expressions with Fractional Exponents

When simplifying exponential expressions with fractional exponents, you can rewrite the expression as a root. For example, 2^(1/2) can be rewritten as √2. This rule applies to complex fractions as well: 3^(2/3) can be rewritten as ∛(3^2).

Examples:

• 2^(1/2) = √2
• 3^(2/3) = ∛(3^2) = ∛9
• 4^(3/4) = 4^(3/4) = ∜(4^3)

Method 5: Simplifying Exponential Expressions with Rational Exponents

When simplifying exponential expressions with rational exponents, you can rewrite the expression as a combination of integer and fractional exponents. For example, 2^(3/2) can be rewritten as 2^3 × 2^(1/2). This rule applies to complex expressions as well: 3^(4/3) can be rewritten as 3^4 × 3^(-1/3).

Examples:

• 2^(3/2) = 2^3 × 2^(1/2) = 8 × √2
• 3^(4/3) = 3^4 × 3^(-1/3) = 81 × ∛(1/3)
• 4^(2/5) = 4^2 × 4^(-3/5) = 16 × ∜(1/4)

In conclusion, simplifying exponential expressions requires a combination of rules and strategies. By understanding the properties of exponents and applying the five methods outlined in this article, you can simplify even the most complex exponential expressions with ease.