Convert Ellipse To Standard Form Easily

Quick Links :

Understanding Ellipses in Mathematics

In mathematics, an ellipse is a type of curve that is closed and has a symmetrical shape. It is defined as the set of all points in a plane such that the sum of their distances from two fixed points (called foci) is constant. Ellipses are used to describe various phenomena in physics, engineering, and other fields, making them an essential concept to grasp. Converting an ellipse to its standard form is a crucial step in working with ellipses, and in this article, we will explore how to do it easily.

What is the Standard Form of an Ellipse?

The standard form of an ellipse is an equation that represents the ellipse in a specific format. This format is used to simplify calculations and make it easier to work with ellipses. The standard form of an ellipse is:

(x - h)^2/a^2 + (y - k)^2/b^2 = 1

where:

• (h, k) is the center of the ellipse
• a is the semi-major axis (the distance from the center to the farthest point on the ellipse)
• b is the semi-minor axis (the distance from the center to the closest point on the ellipse)

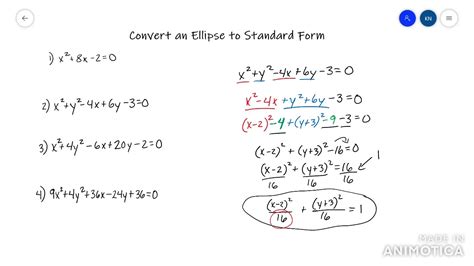
How to Convert an Ellipse to Standard Form

Converting an ellipse to its standard form involves several steps. Here is a step-by-step guide:

1. Identify the center of the ellipse: The center of the ellipse is the point (h, k) that is equidistant from the two foci.
2. Determine the semi-major axis (a): The semi-major axis is the distance from the center to the farthest point on the ellipse.
3. Determine the semi-minor axis (b): The semi-minor axis is the distance from the center to the closest point on the ellipse.
4. Write the equation in standard form: Using the values obtained in steps 1-3, write the equation of the ellipse in standard form.

Examples of Converting Ellipses to Standard Form

Here are a few examples of converting ellipses to standard form:

Example 1: Convert the equation x^2/16 + y^2/9 = 1 to standard form.

Solution: The center of the ellipse is (0, 0), the semi-major axis is 4, and the semi-minor axis is 3. The equation in standard form is:

(x - 0)^2/4^2 + (y - 0)^2/3^2 = 1

Example 2: Convert the equation (x + 2)^2/25 + (y - 3)^2/16 = 1 to standard form.

Solution: The center of the ellipse is (-2, 3), the semi-major axis is 5, and the semi-minor axis is 4. The equation in standard form is:

(x + 2)^2/5^2 + (y - 3)^2/4^2 = 1

Tips and Tricks for Converting Ellipses to Standard Form

Here are a few tips and tricks to keep in mind when converting ellipses to standard form:

• Always identify the center of the ellipse first.
• Use the equation of the ellipse to determine the semi-major and semi-minor axes.
• Make sure to write the equation in standard form, using the correct format.

Conclusion and Final Thoughts

Converting an ellipse to its standard form is a crucial step in working with ellipses. By following the steps outlined in this article, you can easily convert an ellipse to its standard form. Remember to identify the center of the ellipse, determine the semi-major and semi-minor axes, and write the equation in standard form. With practice and patience, you can become proficient in converting ellipses to standard form.

We hope this article has been helpful in explaining how to convert an ellipse to its standard form. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences in the comments below!

FAQ Section: