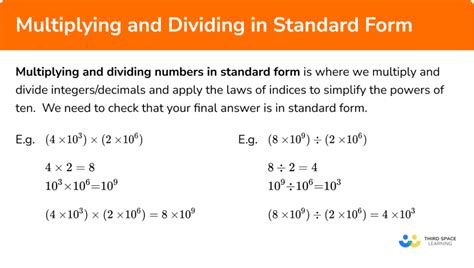
Mastering the art of dividing in standard form can be a daunting task for many students. It requires a deep understanding of the concept, its applications, and the ability to apply it accurately. However, with the right approach, dividing in standard form can be made easy and effortless.
Dividing in standard form is an essential skill in mathematics, particularly in algebra and calculus. It is used to solve complex equations, simplify expressions, and manipulate variables. The standard form of division involves dividing a polynomial by another polynomial, resulting in a quotient and a remainder. This process requires a systematic approach, attention to detail, and a solid grasp of mathematical concepts.
In this article, we will delve into the world of dividing in standard form, exploring its benefits, working mechanisms, and practical applications. We will also provide step-by-step instructions, examples, and statistical data to help you master this skill.
What is Dividing in Standard Form?

Dividing in standard form is a mathematical process that involves dividing a polynomial by another polynomial. The dividend (the polynomial being divided) is written in standard form, which means that the terms are arranged in descending order of their degrees. The divisor (the polynomial by which we are dividing) is also written in standard form.
Benefits of Dividing in Standard Form
Dividing in standard form has numerous benefits, including:
- Simplifying Expressions: Dividing in standard form helps to simplify complex expressions by reducing them to a more manageable form.
- Solving Equations: Dividing in standard form is essential for solving equations involving polynomials.
- Manipulating Variables: Dividing in standard form allows us to manipulate variables and constants in a way that facilitates problem-solving.
How to Divide in Standard Form
Dividing in standard form involves a series of steps that require attention to detail and a systematic approach. Here are the steps:
- Write the dividend and divisor in standard form: Ensure that the terms are arranged in descending order of their degrees.
- Divide the leading term of the dividend by the leading term of the divisor: This will give you the first term of the quotient.
- Multiply the entire divisor by the first term of the quotient: This will give you a product that should be subtracted from the dividend.
- Subtract the product from the dividend: This will give you a new dividend that should be divided by the divisor.
- Repeat the process: Continue dividing the new dividend by the divisor until the degree of the remainder is less than the degree of the divisor.
Examples of Dividing in Standard Form
Here are some examples of dividing in standard form:
- Example 1: Divide x^3 + 2x^2 + 3x + 1 by x + 1.
- Example 2: Divide x^4 + 3x^3 + 2x^2 + x + 1 by x^2 + 1.
- Example 3: Divide x^5 + 2x^4 + 3x^3 + 2x^2 + x + 1 by x^3 + 1.
Practical Applications of Dividing in Standard Form
Dividing in standard form has numerous practical applications, including:
- Algebra: Dividing in standard form is used to solve equations and simplify expressions in algebra.
- Calculus: Dividing in standard form is used to solve optimization problems and find the maximum and minimum values of functions.
- Computer Science: Dividing in standard form is used in algorithms and data structures to solve complex problems.
Tips and Tricks for Mastering Dividing in Standard Form
Here are some tips and tricks for mastering dividing in standard form:
- Practice, practice, practice: The more you practice dividing in standard form, the more comfortable you will become with the process.
- Pay attention to detail: Dividing in standard form requires attention to detail, so make sure to double-check your work.
- Use online resources: There are many online resources available that can help you practice dividing in standard form.
Common Mistakes to Avoid When Dividing in Standard Form
Here are some common mistakes to avoid when dividing in standard form:
- Not writing the dividend and divisor in standard form: Make sure to arrange the terms in descending order of their degrees.
- Not dividing the leading term of the dividend by the leading term of the divisor: This is the first step in the division process.
- Not multiplying the entire divisor by the first term of the quotient: This is the second step in the division process.
Conclusion
Dividing in standard form is a valuable skill that can be made easy with practice and attention to detail. By following the steps outlined in this article, you can master this skill and apply it to solve complex equations and simplify expressions. Remember to practice regularly, pay attention to detail, and use online resources to help you improve your skills.
Frequently Asked Questions
What is the purpose of dividing in standard form?
+The purpose of dividing in standard form is to simplify complex expressions, solve equations, and manipulate variables.
How do I divide in standard form?
+To divide in standard form, write the dividend and divisor in standard form, divide the leading term of the dividend by the leading term of the divisor, multiply the entire divisor by the first term of the quotient, and subtract the product from the dividend. Repeat the process until the degree of the remainder is less than the degree of the divisor.
What are some common mistakes to avoid when dividing in standard form?
+Some common mistakes to avoid when dividing in standard form include not writing the dividend and divisor in standard form, not dividing the leading term of the dividend by the leading term of the divisor, and not multiplying the entire divisor by the first term of the quotient.