3 Ways To Convert Standard Form To Vertex Form

Quick Links :

Converting standard form to vertex form is a crucial skill in mathematics, particularly in algebra and graphing. The vertex form of a quadratic function is a powerful tool for analyzing and understanding the behavior of parabolas. In this article, we will explore three ways to convert standard form to vertex form, providing you with a comprehensive understanding of the process.

Understanding Standard Form and Vertex Form

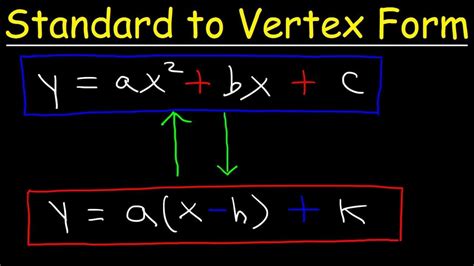
Standard form, also known as general form, is a way of writing a quadratic function in the form of ax^2 + bx + c = 0, where a, b, and c are constants. On the other hand, vertex form is a way of writing a quadratic function in the form of a(x - h)^2 + k, where (h, k) represents the coordinates of the vertex of the parabola.

The Importance of Vertex Form

Vertex form is essential in graphing and analyzing quadratic functions. It provides valuable information about the parabola, such as the coordinates of the vertex, the axis of symmetry, and the direction of opening. By converting standard form to vertex form, you can easily identify the key features of the parabola and make informed decisions about its behavior.

Method 1: Completing the Square

Completing the square is a popular method for converting standard form to vertex form. This method involves manipulating the quadratic expression to create a perfect square trinomial.

To complete the square, follow these steps:

1. Factor out the coefficient of x^2 from the quadratic expression.
2. Divide the coefficient of x by 2 and square the result.
3. Add and subtract the squared result within the parentheses.
4. Factor the perfect square trinomial and simplify.

Example 1: Completing the Square

Convert the standard form quadratic function x^2 + 6x + 8 = 0 to vertex form using completing the square.

1. Factor out the coefficient of x^2: x^2 + 6x + 8 = (x^2 + 6x) + 8
2. Divide the coefficient of x by 2 and square the result: (6/2)^2 = 9
3. Add and subtract 9 within the parentheses: (x^2 + 6x + 9 - 9) + 8
4. Factor the perfect square trinomial and simplify: (x + 3)^2 - 1

The vertex form of the quadratic function is (x + 3)^2 - 1.

Method 2: Using the Vertex Formula

Another method for converting standard form to vertex form is by using the vertex formula. The vertex formula states that for a quadratic function in the form ax^2 + bx + c, the coordinates of the vertex are given by:

h = -b / 2a k = c - (b^2 / 4a)

To use the vertex formula, follow these steps:

1. Identify the values of a, b, and c in the standard form quadratic function.
2. Calculate the x-coordinate of the vertex using the formula h = -b / 2a.
3. Calculate the y-coordinate of the vertex using the formula k = c - (b^2 / 4a).
4. Write the vertex form of the quadratic function using the coordinates of the vertex.

Example 2: Using the Vertex Formula

Convert the standard form quadratic function x^2 + 4x + 5 = 0 to vertex form using the vertex formula.

1. Identify the values of a, b, and c: a = 1, b = 4, c = 5
2. Calculate the x-coordinate of the vertex: h = -4 / (2 * 1) = -2
3. Calculate the y-coordinate of the vertex: k = 5 - (4^2 / (4 * 1)) = 5 - 4 = 1
4. Write the vertex form of the quadratic function: (x + 2)^2 + 1

The vertex form of the quadratic function is (x + 2)^2 + 1.

Method 3: Using Graphing Calculators

Graphing calculators can also be used to convert standard form to vertex form. Most graphing calculators have a built-in feature that allows you to enter a quadratic function in standard form and display its vertex form.

To use a graphing calculator, follow these steps:

1. Enter the standard form quadratic function into the calculator.
2. Use the calculator's built-in feature to display the vertex form of the quadratic function.
3. Record the vertex form of the quadratic function.

Example 3: Using Graphing Calculators

Convert the standard form quadratic function x^2 + 3x + 2 = 0 to vertex form using a graphing calculator.

1. Enter the standard form quadratic function into the calculator: x^2 + 3x + 2 = 0
2. Use the calculator's built-in feature to display the vertex form: (x + 1.5)^2 - 0.25
3. Record the vertex form of the quadratic function: (x + 1.5)^2 - 0.25

The vertex form of the quadratic function is (x + 1.5)^2 - 0.25.

Conclusion

Converting standard form to vertex form is an essential skill in mathematics. In this article, we have explored three methods for converting standard form to vertex form: completing the square, using the vertex formula, and using graphing calculators. Each method has its own advantages and disadvantages, and it's essential to understand the strengths and weaknesses of each approach.

By mastering these methods, you can convert standard form to vertex form with ease and analyze quadratic functions with confidence. Remember to practice each method and apply them to different problems to reinforce your understanding.