3 Ways To Convert To Vertex Form By Completing Square

Quick Links :

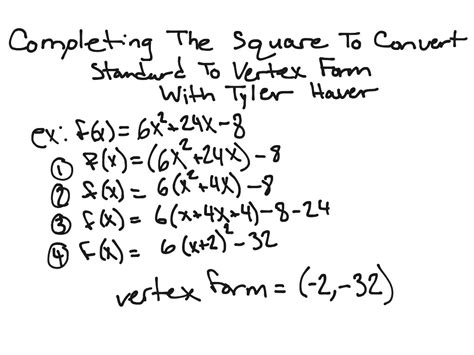
Completing the square is a fundamental concept in algebra that allows us to convert a quadratic function from its standard form to vertex form. This process is essential for graphing quadratic functions and understanding their properties. In this article, we will explore three ways to convert a quadratic function to vertex form by completing the square.

Understanding the Importance of Vertex Form

Vertex form is a way of expressing a quadratic function in the form f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. This form is useful for graphing quadratic functions, as it provides us with the coordinates of the vertex and the direction of the parabola's opening. By converting a quadratic function to vertex form, we can easily identify the vertex, axis of symmetry, and the direction of the parabola's opening.

Method 1: Completing the Square for Quadratic Functions with a Leading Coefficient of 1

When the leading coefficient of a quadratic function is 1, we can complete the square by following these steps:

• Write the quadratic function in standard form: f(x) = x^2 + bx + c
• Move the constant term to the right-hand side: f(x) = x^2 + bx = -c
• Add (b/2)^2 to both sides: f(x) = x^2 + bx + (b/2)^2 = -c + (b/2)^2
• Factor the left-hand side: f(x) = (x + b/2)^2 = -c + (b/2)^2
• Simplify the right-hand side: f(x) = (x + b/2)^2 = -(c - (b/2)^2)

For example, let's convert the quadratic function f(x) = x^2 + 6x + 8 to vertex form:

f(x) = x^2 + 6x = -8 f(x) = x^2 + 6x + 9 = -8 + 9 f(x) = (x + 3)^2 = 1

So, the vertex form of the quadratic function is f(x) = (x + 3)^2 + 1.

Method 1 Example: Converting a Quadratic Function to Vertex Form

Let's convert the quadratic function f(x) = x^2 + 4x + 3 to vertex form using Method 1:

f(x) = x^2 + 4x = -3 f(x) = x^2 + 4x + 4 = -3 + 4 f(x) = (x + 2)^2 = 1

So, the vertex form of the quadratic function is f(x) = (x + 2)^2 + 1.

Method 2: Completing the Square for Quadratic Functions with a Leading Coefficient Other Than 1

When the leading coefficient of a quadratic function is not 1, we need to factor out the leading coefficient before completing the square:

• Write the quadratic function in standard form: f(x) = ax^2 + bx + c
• Factor out the leading coefficient: f(x) = a(x^2 + (b/a)x + c/a)
• Move the constant term to the right-hand side: f(x) = a(x^2 + (b/a)x) = -ac/a
• Add (b/2a)^2 to both sides: f(x) = a(x^2 + (b/a)x + (b/2a)^2) = -ac/a + (b/2a)^2
• Factor the left-hand side: f(x) = a(x + b/2a)^2 = -ac/a + (b/2a)^2
• Simplify the right-hand side: f(x) = a(x + b/2a)^2 = -(ac - (b/2a)^2)/a

For example, let's convert the quadratic function f(x) = 2x^2 + 12x + 18 to vertex form:

f(x) = 2(x^2 + 6x) = -18 f(x) = 2(x^2 + 6x + 9) = -18 + 18 f(x) = 2(x + 3)^2 = 0

So, the vertex form of the quadratic function is f(x) = 2(x + 3)^2.

Method 2 Example: Converting a Quadratic Function to Vertex Form

Let's convert the quadratic function f(x) = 3x^2 + 12x + 15 to vertex form using Method 2:

f(x) = 3(x^2 + 4x) = -15 f(x) = 3(x^2 + 4x + 4) = -15 + 12 f(x) = 3(x + 2)^2 = -3

So, the vertex form of the quadratic function is f(x) = 3(x + 2)^2 - 3.

Method 3: Using the Vertex Form Formula

Another way to convert a quadratic function to vertex form is by using the vertex form formula:

h = -b/2a k = f(h)

where (h, k) is the vertex of the parabola.

For example, let's convert the quadratic function f(x) = x^2 + 6x + 8 to vertex form using the vertex form formula:

h = -6/2(1) = -3 k = f(-3) = (-3)^2 + 6(-3) + 8 = 1

So, the vertex form of the quadratic function is f(x) = (x + 3)^2 + 1.

Method 3 Example: Converting a Quadratic Function to Vertex Form

Let's convert the quadratic function f(x) = 2x^2 + 12x + 18 to vertex form using Method 3:

h = -12/2(2) = -3 k = f(-3) = 2(-3)^2 + 12(-3) + 18 = 0

So, the vertex form of the quadratic function is f(x) = 2(x + 3)^2.

In conclusion, completing the square is an essential technique for converting a quadratic function to vertex form. By using one of the three methods outlined in this article, you can easily convert a quadratic function to vertex form and gain a deeper understanding of its properties.

We hope this article has helped you understand the importance of vertex form in quadratic functions and the three methods for completing the square. If you have any questions or need further clarification, please don't hesitate to ask.