Converting to radical form can seem daunting, especially for students who are new to algebra. However, with the right techniques and strategies, it can be a breeze. In this article, we will explore three ways to convert to radical form with ease, along with practical examples and step-by-step explanations.
Understanding the Basics of Radical Form

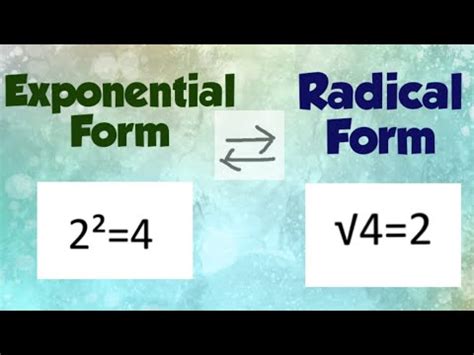
Before we dive into the methods of converting to radical form, it's essential to understand the basics. Radical form, also known as rational exponent form, is a way of expressing a number with a fractional exponent. For example, √(x) can be written as x^(1/2). The key concept to grasp is that the denominator of the fraction represents the root, while the numerator represents the power.
The Importance of Converting to Radical Form
Converting to radical form is crucial in algebra, as it allows for easier manipulation and simplification of expressions. It's also a fundamental skill required for solving equations and inequalities. By mastering the techniques of converting to radical form, students can improve their problem-solving skills and build a stronger foundation in algebra.
Method 1: Using the Definition of Radical Form

The first method involves using the definition of radical form to convert expressions. According to the definition, x^(m/n) = √(x^m). Using this definition, we can convert expressions from rational exponent form to radical form.
Example: Convert x^(2/3) to radical form.
Step 1: Identify the numerator (2) and denominator (3) of the fraction. Step 2: Apply the definition of radical form: x^(2/3) = √(x^2) Step 3: Simplify the expression: x^(2/3) = √(x^2) = (√x)^2
Method 2: Using the Properties of Exponents
Another method of converting to radical form involves using the properties of exponents. Specifically, the property x^(m/n) = (x^m)^(1/n) can be used to convert expressions.
Example: Convert x^(3/4) to radical form.
Step 1: Apply the property: x^(3/4) = (x^3)^(1/4) Step 2: Simplify the expression: x^(3/4) = (x^3)^(1/4) = ⁴√(x^3)
Method 3: Using the Relationship Between Radical Form and Rational Exponents

The third method involves using the relationship between radical form and rational exponents. Specifically, the relationship x^(m/n) = √(x^m) can be used to convert expressions.
Example: Convert x^(4/5) to radical form.
Step 1: Identify the numerator (4) and denominator (5) of the fraction. Step 2: Apply the relationship: x^(4/5) = √(x^4) Step 3: Simplify the expression: x^(4/5) = √(x^4) = (√x)^4
Conclusion
Converting to radical form is an essential skill in algebra, and mastering the techniques can make a huge difference in problem-solving. By using the definition of radical form, properties of exponents, and the relationship between radical form and rational exponents, students can convert expressions with ease. Remember to practice, practice, practice, and you'll be a pro at converting to radical form in no time!
What is radical form?
+Radical form, also known as rational exponent form, is a way of expressing a number with a fractional exponent.
Why is converting to radical form important?
+Converting to radical form is crucial in algebra, as it allows for easier manipulation and simplification of expressions.
What are some common methods of converting to radical form?
+Common methods include using the definition of radical form, properties of exponents, and the relationship between radical form and rational exponents.