Convert Circle Equations To Standard Form Easily

Quick Links :

Learning to convert circle equations to standard form is a fundamental concept in mathematics, particularly in algebra and geometry. Understanding how to express circle equations in standard form can simplify many problems and make it easier to analyze and visualize the properties of circles.

Understanding Circle Equations

A circle equation represents the relationship between the coordinates of points on a circle and the center of the circle. The general form of a circle equation is (x - h)^2 + (y - k)^2 = r^2, where (h, k) is the center of the circle and r is the radius. However, not all circle equations are given in this standard form. To analyze and work with circle equations, it's essential to know how to convert them to standard form.

Why Convert to Standard Form?

Converting circle equations to standard form is crucial for several reasons:

• Simplifies the equation: Standard form makes it easier to identify the center and radius of the circle, which are essential parameters in many mathematical and real-world problems.
• Facilitates analysis: Standard form enables us to analyze the properties of the circle, such as its position, size, and orientation.
• Enhances visualization: By expressing the circle equation in standard form, we can better visualize the circle and its relationship with other geometric shapes.

Steps to Convert Circle Equations to Standard Form

Converting a circle equation to standard form involves a series of steps:

Step 1: Identify the General Form

The first step is to identify the general form of the circle equation, which is (x - h)^2 + (y - k)^2 = r^2.

Step 2: Rearrange the Equation

Rearrange the equation to isolate the squared terms on one side:

(x^2 - 2hx + h^2) + (y^2 - 2ky + k^2) = r^2

Step 3: Complete the Square

Complete the square for both x and y terms:

(x^2 - 2hx + h^2) + (y^2 - 2ky + k^2) = r^2

(x - h)^2 + (y - k)^2 = r^2

Step 4: Identify the Center and Radius

Identify the center (h, k) and radius r from the standard form equation.

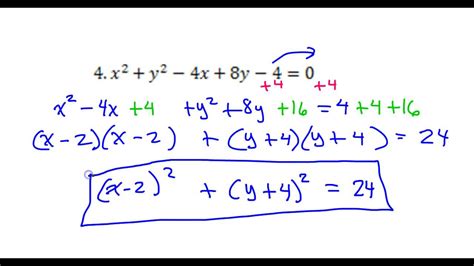
Example 1: Converting a Circle Equation to Standard Form

Suppose we have the circle equation:

x^2 + y^2 + 4x + 6y + 12 = 0

To convert this equation to standard form, follow the steps above:

Rearrange the equation:

(x^2 + 4x) + (y^2 + 6y) = -12

Complete the square:

(x^2 + 4x + 4) + (y^2 + 6y + 9) = -12 + 4 + 9

(x + 2)^2 + (y + 3)^2 = 1

Identify the center and radius:

Center: (-2, -3) Radius: 1

Challenges and Tips

Converting circle equations to standard form can be challenging, especially when dealing with complex equations. Here are some tips to help you overcome common challenges:

• Check your calculations: Double-check your calculations to ensure accuracy.
• Use algebraic manipulations: Use algebraic manipulations, such as completing the square, to simplify the equation.
• Visualize the circle: Visualize the circle to ensure that the standard form equation makes sense.

Real-World Applications

Converting circle equations to standard form has numerous real-world applications:

• Engineering: Circle equations are used to design and analyze circular structures, such as bridges, tunnels, and pipes.
• Computer Science: Circle equations are used in computer graphics, game development, and geometric modeling.
• Physics: Circle equations are used to describe the motion of objects in circular orbits, such as planets and satellites.

Conclusion: Mastering Circle Equations

Mastering the skill of converting circle equations to standard form is essential for anyone working with circles in mathematics, science, and engineering. By following the steps outlined above and practicing with examples, you can develop a deep understanding of circle equations and their applications.