7e11 In Standard Form: Simplified Explanation And Conversion

Quick Links :

The world of numbers can be fascinating, and understanding standard form is a crucial part of it. In this article, we will delve into the concept of 7e11 in standard form, explaining what it means and how to convert it into a more familiar format.

What is Standard Form?

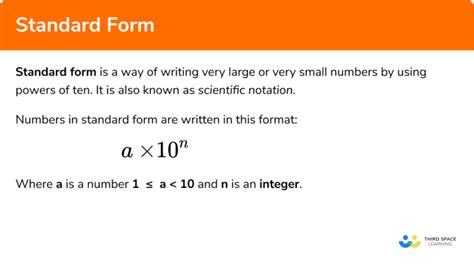
Standard form, also known as scientific notation, is a way of expressing numbers in a more compact and readable format. It is commonly used in mathematics, physics, and engineering to represent very large or very small numbers. The standard form of a number is written in the form:

a × 10^n

where 'a' is a number between 1 and 10, and 'n' is an integer.

Why is Standard Form Useful?

Standard form is useful because it allows us to easily represent and manipulate very large or very small numbers. It also helps to avoid errors that can occur when working with numbers in their standard decimal form.

What Does 7e11 Mean?

7e11 is a number written in standard form. The '7' represents the coefficient, and the 'e11' represents the exponent. In this case, the 'e' stands for 'exponent' and is equivalent to '× 10^'. Therefore, 7e11 can be written as:

7 × 10^11

Converting 7e11 to Standard Decimal Form

To convert 7e11 to standard decimal form, we need to multiply the coefficient (7) by 10 raised to the power of the exponent (11).

7 × 10^11 = 70,000,000,000

So, 7e11 is equal to 70 billion in standard decimal form.

Real-World Applications of Standard Form

Standard form has many real-world applications in fields such as physics, engineering, and computer science. It is used to represent quantities such as:

• Very large numbers, such as the number of atoms in a mole (6.022 × 10^23)
• Very small numbers, such as the size of a bacterium (0.00005 meters)
• Quantities with a large range, such as the distance from the Earth to the Sun (1.496 × 10^11 meters)

Common Examples of Standard Form

Here are a few more examples of numbers written in standard form:

• 3.2 × 10^4 (32,000)
• 2.5 × 10^-3 (0.0025)
• 1.8 × 10^6 (1,800,000)

Converting Numbers to Standard Form

To convert a number to standard form, follow these steps:

1. Move the decimal point to the left or right until you have a number between 1 and 10.
2. Count the number of places you moved the decimal point.
3. Write the number in the form a × 10^n, where 'a' is the number between 1 and 10, and 'n' is the number of places you moved the decimal point.

For example, to convert 456,000 to standard form:

1. Move the decimal point 5 places to the left to get 4.56.
2. Write the number in the form 4.56 × 10^5.

Practice Exercises

Try converting the following numbers to standard form:

• 234,000
• 0.000045
• 987,654,321

Check your answers by converting the numbers back to standard decimal form.

Conclusion

Standard form is an essential concept in mathematics and science, allowing us to easily represent and manipulate very large or very small numbers. By understanding how to convert numbers to and from standard form, you can simplify complex calculations and improve your problem-solving skills. We hope this article has helped you understand the concept of 7e11 in standard form and how to work with numbers in this format.