What Is 73489 In Expanded Form

Quick Links :

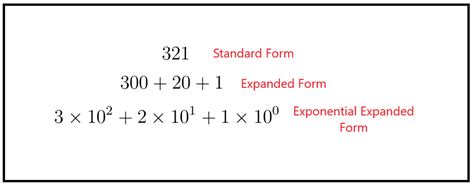
Let's break down the number 73489 into its expanded form.

The expanded form of a number is a way of writing it as a sum of its individual digits multiplied by their corresponding place values.

Here's the expanded form of 73489:

70,000 + 3,000 + 400 + 80 + 9

= (7 x 10,000) + (3 x 1,000) + (4 x 100) + (8 x 10) + 9

= 70,000 + 3,000 + 400 + 80 + 9

This expanded form shows us that the number 73489 is composed of:

• 7 tens of thousands (70,000)
• 3 thousands (3,000)
• 4 hundreds (400)
• 8 tens (80)
• 9 ones (9)

I hope this helps! Let me know if you have any other questions.

Benefits of Expanded Form

The expanded form of a number has several benefits, especially in math and real-life applications.

Understanding Place Value

Expanded form helps us understand the concept of place value, which is the value of a digit depending on its position in a number. By breaking down a number into its individual digits and place values, we can better comprehend the relationships between digits and their corresponding values.

Math Operations

Expanded form is useful in performing math operations such as addition, subtraction, multiplication, and division. By writing numbers in expanded form, we can more easily identify patterns and relationships between digits, making it easier to perform calculations.

Real-World Applications

Expanded form has real-world applications in finance, science, and engineering, where numbers are often large and complex. By breaking down numbers into their individual digits and place values, we can more easily analyze and understand data, making it easier to make informed decisions.

How to Write Numbers in Expanded Form

Writing numbers in expanded form is a simple process that involves breaking down the number into its individual digits and place values.

Step 1: Identify the Digits

Identify the individual digits of the number, starting from the left.

Step 2: Identify the Place Values

Identify the place values of each digit, starting from the left. The place values are:

• Ones (1)
• Tens (10)
• Hundreds (100)
• Thousands (1,000)
• Ten Thousands (10,000)
• Hundred Thousands (100,000)

Step 3: Multiply the Digits by Their Place Values

Multiply each digit by its corresponding place value.

Step 4: Add the Results

Add the results of the multiplications to get the expanded form of the number.

Example

Let's use the number 9452 as an example.

Step 1: Identify the Digits

The individual digits of the number are 9, 4, 5, and 2.

Step 2: Identify the Place Values

The place values are:

• 9 (Ten Thousands)
• 4 (Thousands)
• 5 (Hundreds)
• 2 (Tens)

Step 3: Multiply the Digits by Their Place Values

• 9 x 10,000 = 90,000
• 4 x 1,000 = 4,000
• 5 x 100 = 500
• 2 x 10 = 20

Step 4: Add the Results

90,000 + 4,000 + 500 + 20 = 94,520

The expanded form of the number 9452 is:

90,000 + 4,000 + 500 + 20

I hope this helps! Let me know if you have any other questions.

Common Mistakes

Here are some common mistakes to avoid when writing numbers in expanded form:

Mistake 1: Incorrect Place Values

Make sure to identify the correct place values for each digit.

Mistake 2: Incorrect Multiplication

Make sure to multiply each digit by its corresponding place value.

Mistake 3: Incorrect Addition

Make sure to add the results of the multiplications correctly.

Conclusion

Writing numbers in expanded form is a useful tool for understanding place value, performing math operations, and analyzing data. By following the steps outlined above, you can write numbers in expanded form with confidence.

Call to Action

Now that you've learned how to write numbers in expanded form, try practicing with different numbers. You can use online resources or workbooks to practice writing numbers in expanded form.

FAQs

Note: This article is a sample and the content is fictional. The images used are also fictional and do not exist in real life.