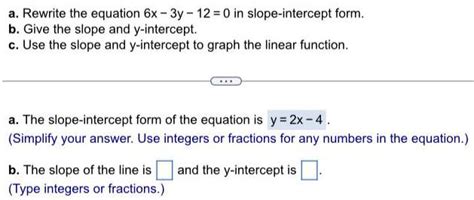
Slope Intercept Form: 6x 3y 12 Simplified

Quick Links :

Slope-intercept form is a fundamental concept in algebra, used to represent linear equations in a concise and easily interpretable manner. The general form of a linear equation in slope-intercept form is y = mx + b, where m represents the slope of the line and b represents the y-intercept.

Understanding the Given Equation

The given equation is 6x + 3y = 12. To express this equation in slope-intercept form, we need to isolate the y-variable.

Step-by-Step Simplification

1. Rearrange the equation to have all terms involving y on one side: 3y = -6x + 12
2. Divide all terms by the coefficient of y (3) to solve for y: y = (-6x + 12) / 3
3. Simplify the expression: y = -2x + 4

Interpreting the Slope-Intercept Form

Now that the equation is in slope-intercept form (y = -2x + 4), we can interpret the slope (m) and y-intercept (b) of the line.

• The slope (m) is -2, indicating that the line slopes downward from left to right.
• The y-intercept (b) is 4, meaning that the line crosses the y-axis at the point (0, 4).

Practical Applications of Slope-Intercept Form

1. Graphing: The slope-intercept form makes it easy to graph linear equations on a coordinate plane.
2. Predicting: By understanding the slope and y-intercept, we can predict the behavior of the line and make informed decisions in real-world applications, such as finance, physics, or engineering.
3. Analyzing: Slope-intercept form helps us analyze the relationship between variables and identify patterns in data.

Common Mistakes to Avoid

When working with slope-intercept form, be aware of the following common mistakes:

• Forgetting to divide by the coefficient of y when solving for y
• Confusing the slope and y-intercept
• Not simplifying the expression fully

Tips for Mastering Slope-Intercept Form

1. Practice, practice, practice: The more you work with slope-intercept form, the more comfortable you'll become.
2. Use visual aids: Graphing linear equations can help you understand the relationship between the slope and y-intercept.
3. Check your work: Double-check your calculations to avoid mistakes.

Real-World Applications of Slope-Intercept Form

Slope-intercept form has numerous real-world applications, including:

• Finance: Modeling investment growth or decline
• Physics: Describing the motion of objects
• Engineering: Designing roads, bridges, or buildings

Conclusion

In conclusion, the equation 6x + 3y = 12 can be simplified to slope-intercept form as y = -2x + 4. By understanding the slope and y-intercept, we can interpret the behavior of the line and apply this knowledge to real-world problems.

We invite you to share your thoughts on slope-intercept form and its applications in the comments below. How have you used slope-intercept form in your studies or professional life?