33 As A Fraction In Simplest Form

Quick Links :

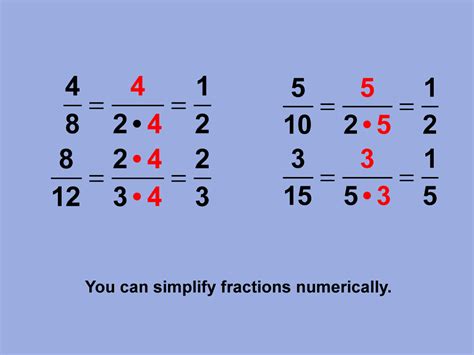
Understanding fractions is a fundamental concept in mathematics, and simplifying them is an essential skill to master. In this article, we'll delve into the world of fractions, specifically focusing on the number 33 as a fraction in its simplest form.

What are Fractions?

Fractions are mathematical expressions that represent a part of a whole. They consist of two main components: the numerator and the denominator. The numerator tells us how many equal parts we have, while the denominator indicates how many parts the whole is divided into. For example, in the fraction 1/2, the numerator is 1, and the denominator is 2.

Why Simplify Fractions?

Simplifying fractions is crucial because it helps us understand the relationship between the numerator and the denominator more clearly. When we simplify a fraction, we reduce it to its most basic form, making it easier to work with and compare to other fractions.

How to Simplify Fractions

To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and the denominator. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder. Once we find the GCD, we can divide both the numerator and the denominator by this number to simplify the fraction.

33 as a Fraction in Simplest Form

Now, let's find 33 as a fraction in its simplest form. Since 33 is a whole number, we can express it as a fraction with a denominator of 1: 33/1. However, this is not the simplest form, as we can simplify it further.

To simplify 33/1, we need to find the GCD of 33 and 1. The GCD of 33 and 1 is 1, as 1 is the only number that divides both 33 and 1 without leaving a remainder. Therefore, the simplest form of 33 as a fraction is still 33/1.

However, if we consider the fraction 33/3, we can simplify it further. The GCD of 33 and 3 is 3, as 3 is the largest number that divides both 33 and 3 without leaving a remainder. Dividing both the numerator and the denominator by 3, we get:

33 ÷ 3 = 11 3 ÷ 3 = 1

So, the simplest form of 33/3 is 11/1.

Benefits of Simplifying Fractions

Simplifying fractions has numerous benefits in mathematics and real-life applications. Some of the advantages include:

• Easier calculations: Simplified fractions make calculations more manageable and less prone to errors.
• Improved understanding: Simplifying fractions helps us comprehend the relationship between the numerator and the denominator more clearly.
• Enhanced comparison: Simplified fractions enable us to compare and order fractions more efficiently.

Real-World Applications of Simplifying Fractions

Simplifying fractions has numerous real-world applications, including:

• Cooking and recipes: Simplified fractions help us measure ingredients accurately and scale recipes up or down.
• Finance and banking: Simplified fractions are used in interest rates, investment calculations, and financial modeling.
• Science and engineering: Simplified fractions are essential in calculations involving proportions, ratios, and percentages.

Conclusion and Next Steps

In conclusion, simplifying fractions is an essential skill in mathematics, and understanding 33 as a fraction in its simplest form is a fundamental concept. By mastering the art of simplifying fractions, we can improve our calculations, comparisons, and real-world applications. To further develop your skills, practice simplifying different fractions, explore real-world examples, and experiment with various mathematical operations involving fractions.

We hope this article has provided you with a comprehensive understanding of 33 as a fraction in its simplest form. If you have any questions or topics you'd like to discuss, please share your thoughts in the comments below.