Convert.3125 To Fraction Form Made Easy

Quick Links :

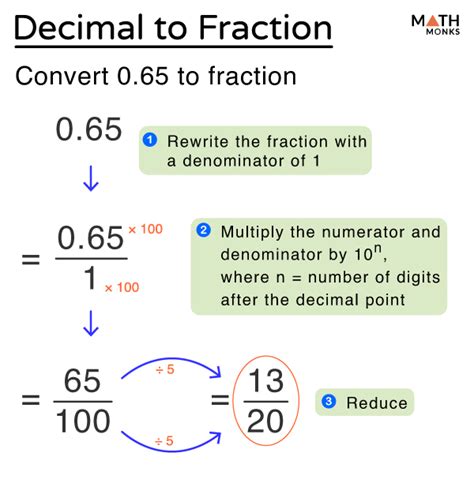
Converting decimals to fractions is a fundamental math concept that can be a bit tricky at times. However, with the right approach, it can be made easy. In this article, we'll explore the process of converting the decimal 0.3125 to its fraction form.

Understanding the Basics

To convert a decimal to a fraction, we need to understand the concept of place value. The decimal point separates the whole number part from the fractional part. The digits after the decimal point represent the fractional part. In the case of 0.3125, we have three digits after the decimal point, which means we're dealing with a fraction that has a denominator of 1000 (10^3).

Method 1: Using the Place Value Approach

One way to convert 0.3125 to a fraction is to use the place value approach. This involves writing the decimal as a fraction with the decimal part as the numerator and the place value as the denominator.

0.3125 = 3125/10000

Now, we can simplify this fraction by dividing both the numerator and the denominator by their greatest common divisor (GCD).

Simplifying the Fraction

To simplify the fraction 3125/10000, we need to find the GCD of the numerator and the denominator. In this case, the GCD is 125.

3125 ÷ 125 = 25 10000 ÷ 125 = 80

So, the simplified fraction is:

0.3125 = 25/80

Method 2: Using Equivalent Fractions

Another way to convert 0.3125 to a fraction is to use equivalent fractions. This involves finding a fraction that has the same value as the original decimal but with a different denominator.

0.3125 = 3125/10000

We can simplify this fraction by finding an equivalent fraction with a smaller denominator.

3125 ÷ 5 = 625 10000 ÷ 5 = 2000

So, the equivalent fraction is:

0.3125 = 625/2000

Further Simplification

We can further simplify the fraction 625/2000 by finding the GCD of the numerator and the denominator. In this case, the GCD is 25.

625 ÷ 25 = 25 2000 ÷ 25 = 80

So, the simplified fraction is:

0.3125 = 25/80

Conclusion

Converting the decimal 0.3125 to its fraction form can be made easy by using the place value approach or equivalent fractions. By simplifying the fraction, we can express 0.3125 as 25/80. This fraction can be used in various mathematical calculations and applications.

Common Applications

The fraction 25/80 has various applications in mathematics, physics, and engineering. For example, it can be used to represent probabilities, proportions, and ratios.

Real-World Examples

1. Probability: The fraction 25/80 can be used to represent the probability of an event occurring. For example, if a bag contains 25 red balls and 80 total balls, the probability of drawing a red ball is 25/80.
2. Proportion: The fraction 25/80 can be used to represent proportions in recipes, scaling, and geometry. For example, if a recipe requires 25 cups of flour and 80 cups of total ingredients, the proportion of flour to total ingredients is 25/80.
3. Ratio: The fraction 25/80 can be used to represent ratios in physics, engineering, and architecture. For example, if a building has a height of 25 meters and a width of 80 meters, the ratio of height to width is 25/80.

Conclusion

In conclusion, converting the decimal 0.3125 to its fraction form can be made easy by using the place value approach or equivalent fractions. The resulting fraction, 25/80, has various applications in mathematics, physics, and engineering. By understanding the concept of place value and simplifying fractions, we can master the art of converting decimals to fractions.

What's Next?

Now that you've learned how to convert 0.3125 to its fraction form, try practicing with other decimals. You can use online calculators or worksheets to practice converting decimals to fractions. Remember to simplify your fractions and apply them to real-world problems.

Take the Next Step

Take the next step in mastering fractions by learning about:

• Equivalent ratios: Learn how to find equivalent ratios and apply them to real-world problems.
• Fractional arithmetic: Practice adding, subtracting, multiplying, and dividing fractions with different denominators.
• Fractional word problems: Learn how to solve word problems involving fractions in various contexts.

By mastering fractions, you'll become proficient in solving mathematical problems and applying them to real-world situations.