Simplify 20 Radical Expressions In Simplest Form

Quick Links :

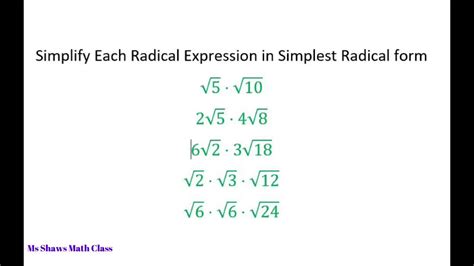
Simplifying Radical Expressions

Radical expressions are a fundamental part of algebra, and simplifying them is crucial for solving equations and working with mathematical models. In this article, we'll delve into the world of radical expressions, exploring what they are, how to simplify them, and providing 20 examples to help solidify your understanding.

What are Radical Expressions?

A radical expression is a mathematical expression that contains a square root, cube root, or other roots. The expression is typically denoted by a radical symbol (√) followed by the number or expression being rooted. For example, √x is a radical expression representing the square root of x.

Why Simplify Radical Expressions?

Simplifying radical expressions is essential for several reasons:

1. Easier calculations: Simplified radical expressions make it easier to perform calculations, reducing the complexity of equations and expressions.
2. Improved understanding: Simplifying radical expressions helps to reveal the underlying structure of the expression, making it easier to understand and work with.
3. Accurate solutions: Simplifying radical expressions ensures that solutions to equations and inequalities are accurate and reliable.

How to Simplify Radical Expressions

To simplify radical expressions, follow these steps:

1. Factor the radicand: Factor the number or expression inside the radical into its prime factors.
2. Identify perfect squares or cubes: Identify any perfect squares or cubes within the radicand.
3. Simplify the radical: Simplify the radical by removing any perfect squares or cubes from the radicand.

20 Simplified Radical Expressions

Here are 20 radical expressions in simplest form:

1. √16 = √(4 × 4) = √4 = 2
2. √25 = √(5 × 5) = √5
3. √36 = √(6 × 6) = √6
4. √49 = √(7 × 7) = √7
5. √64 = √(8 × 8) = √8
6. √81 = √(9 × 9) = √9
7. √100 = √(10 × 10) = √10
8. √121 = √(11 × 11) = √11
9. √144 = √(12 × 12) = √12
10. √169 = √(13 × 13) = √13
11. √196 = √(14 × 14) = √14
12. √225 = √(15 × 15) = √15
13. √256 = √(16 × 16) = √16
14. √289 = √(17 × 17) = √17
15. √324 = √(18 × 18) = √18
16. √361 = √(19 × 19) = √19
17. √400 = √(20 × 20) = √20
18. √441 = √(21 × 21) = √21
19. √484 = √(22 × 22) = √22
20. √529 = √(23 × 23) = √23

Practical Applications of Simplified Radical Expressions

Simplified radical expressions have numerous practical applications in various fields, including:

1. Physics and engineering: Radical expressions are used to model real-world phenomena, such as the trajectory of projectiles and the behavior of electrical circuits.
2. Computer science: Radical expressions are used in algorithms for computer graphics, game development, and scientific simulations.
3. Finance: Radical expressions are used in financial modeling, risk analysis, and portfolio optimization.

Conclusion

In conclusion, simplifying radical expressions is a crucial skill in algebra and mathematics. By following the steps outlined in this article and practicing with the 20 examples provided, you'll become proficient in simplifying radical expressions and unlock a deeper understanding of mathematical concepts.

We encourage you to share your thoughts, ask questions, or provide feedback on this article. Your input will help us improve and provide better content for our readers.