Simplify Fractions In 4 Easy Steps

Quick Links :

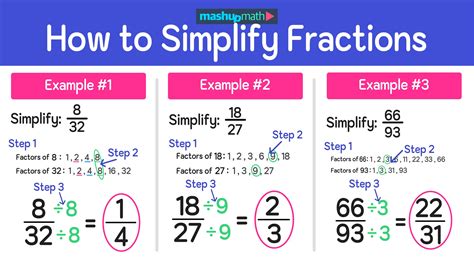
Simplifying fractions is a fundamental math concept that can seem intimidating at first, but with the right approach, it can be a breeze. Fractions are used to represent a part of a whole, and simplifying them involves reducing the numerator and denominator to their simplest form. In this article, we'll break down the process of simplifying fractions into 4 easy steps, making it easy for anyone to master this essential math skill.

Why Simplify Fractions?

Before we dive into the steps, let's quickly discuss why simplifying fractions is important. Simplifying fractions helps to:

• Reduce confusion: Simplified fractions are easier to read and understand, reducing errors and confusion.
• Improve calculations: Simplified fractions make calculations, such as adding and multiplying, much easier.
• Enhance problem-solving: Simplifying fractions can help you solve problems more efficiently and accurately.

Step 1: Find the Greatest Common Divisor (GCD)

The first step in simplifying a fraction is to find the Greatest Common Divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both the numerator and denominator without leaving a remainder.

For example, let's consider the fraction 12/18. To find the GCD, we can list the factors of both numbers:

• Factors of 12: 1, 2, 3, 4, 6, 12
• Factors of 18: 1, 2, 3, 6, 9, 18

The largest number that appears in both lists is 6, so the GCD of 12 and 18 is 6.

How to Find the GCD

There are several ways to find the GCD, including:

• Listing factors: As shown above, list the factors of both numbers and find the largest common factor.
• Using a calculator: Many calculators have a built-in GCD function.
• Using a chart: Create a chart with numbers 1 to 10 and find the largest number that divides both numbers.

Step 2: Divide the Numerator and Denominator by the GCD

Once you've found the GCD, divide both the numerator and denominator by the GCD. This will reduce the fraction to its simplest form.

Using the example from step 1, we can divide both the numerator and denominator by 6:

• Numerator: 12 ÷ 6 = 2
• Denominator: 18 ÷ 6 = 3

So, the simplified fraction is 2/3.

Step 3: Check for Any Remaining Common Factors

After dividing the numerator and denominator by the GCD, check if there are any remaining common factors. If there are, repeat the process of finding the GCD and dividing.

For example, let's consider the fraction 8/12. After dividing by the GCD of 4, we get:

• Numerator: 8 ÷ 4 = 2
• Denominator: 12 ÷ 4 = 3

However, there is still a common factor of 2. So, we repeat the process:

• Numerator: 2 ÷ 2 = 1
• Denominator: 3 ÷ 2 = 1.5 (which is not a whole number, so we can't simplify further)

Step 4: Write the Simplified Fraction

The final step is to write the simplified fraction. Make sure to include the numerator and denominator, separated by a slash.

For example, the simplified fraction from step 2 is 2/3.

Example Problems

Here are a few example problems to practice simplifying fractions:

• Simplify the fraction 15/20.
• Simplify the fraction 24/36.
• Simplify the fraction 9/12.

Take a few minutes to work through these problems, and check your answers against the solutions below:

• 15/20 = 3/4
• 24/36 = 2/3
• 9/12 = 3/4

Conclusion

Simplifying fractions is a straightforward process that can be broken down into 4 easy steps: finding the GCD, dividing the numerator and denominator, checking for remaining common factors, and writing the simplified fraction. By following these steps, you'll be able to simplify fractions with ease and confidence.

What's Next?

Now that you've mastered the art of simplifying fractions, it's time to take your math skills to the next level. Here are a few suggestions for further learning:

• Practice simplifying more complex fractions, such as 48/60 or 120/180.
• Learn about equivalent fractions and how to convert between them.
• Explore other math topics, such as algebra or geometry.

We hope this article has helped you simplify fractions with ease. Share your thoughts and feedback in the comments below, and don't forget to share this article with your friends and family who may need help with simplifying fractions!